-
超构材料是由周期性排列的微纳基元结构组成的人工材料,其基元结构尺寸远小于工作波长。超构材料的特殊光学性质不仅仅源于其材料本身,还可以通过调控其形状、尺寸、方向以及排列方式等自由度,实现操纵电磁波的功能。超构材料可以被用于制造理想透镜(或被称为超级透镜),该透镜允许光学成像的分辨率低于衍射极限。通过阻挡、吸收、增强或弯曲光线,超构材料能够实现传统材料所不能提供的光学功能。超构材料的潜在应用多种多样,包括滤光片、医疗设备、远程航空应用、传感器检测和基础设施监控、智能太阳能管理、天线罩、高增益天线的透镜等等[1-3]。
超构表面是一种具有亚波长厚度的人造片状材料,是超构材料的二维对应物[4-5]。超构表面可以是周期性结构,也可以是非周期性结构。在电磁理论中,超构表面通过特定的边界条件,而不是利用自然材料和三维超构材料中的本构参数来调节电磁波的行为。与超构材料相比,超构表面的加工难度更小,可以有效解决超构材料不可避免的损耗、色散和相位失配等问题。近年来,超构表面已被用于产生多种有趣的光学效应,包括异常反射和透射、平面透镜成像、全息成像、光子自旋霍尔效应和涡旋光束等[6-9]。此外,超构表面在频率转换等非线性光学领域以及量子光学领域也有着广泛的应用前景,有望进一步拓展超构表面的多功能集成能力。
该综述分别总结了近年来超构表面在非线性光学和量子光学的应用,第一部分介绍了非线性光学的概念和基本原理,以及基于超构表面如何实现谐波的产生和增强,讨论了谐波产生和对称性的关系,以及使用超构表面实现非线性相位调控和全息成像;第二部分介绍了等离激元的量子光学效应,以及基于超构表面实现的纠缠光子的产生,测量和调控,最后讨论了超构表面与量子发光体的相互作用。
-
非线性光学是光学的一个分支,它描述了非线性介质中光的行为,其中极化强度对电场强度的响应是非线性的。自然界中的非线性光学效应很微弱,所以需要非常高的光场强度以进行观测,通常使用激光器进行实验。非线性光学解释了诸如频率,偏振,相位或入射光路径等属性的非线性响应。这些非线性相互作用产生了许多非线性光学现象:如频率混合过程(如差频,倍频)、四波混频、光学参量增强、光学参量震荡、光学克尔效应、交叉相位调制、交叉极化波产生等。非线性光学最常用的频率混合过程之一是倍频,或者称为二次谐波产生。利用这种技术,掺钕钇铝石榴石激光器的1 064 nm输出光或掺钛蓝宝石激光器的800 nm输出光可以转换成可见光,波长分别为532 nm (绿色)或400 nm (紫色)。通常认为介质中的分子或者原子在外场激发下产生的非线性极化是其非线性光学效应的微观机制。非线性过程可以用非线性极化强度来描述。材料的电极化强度和入射电磁波的电场满足如下关系:
式中:
${\varepsilon _0}$ 表示真空介电常数;${\chi _1},{\chi _2},{\chi _3}$ 分别表示线性极化率、二阶极化率、三阶级化率。对于自然界常见物质来说,随着阶数增大,其非线性极化率会迅速减小。因此,二阶非线性过程和三阶非线性过程是主要研究的两种非线性过程。常用的二阶非线性光学晶体有磷酸二氢钾、磷酸二氢铵、铌酸钡钠等。非线性超构表面相比于超构材料,其二维属性对材料设计的限制较少,从而有利于生产加工。另一方面,超构材料中的非线性过程通常需要严格地满足相位匹配条件,但是对于具有亚波长厚度的超构表面而言,该条件的限制被大大放宽。 -
为了在纳米尺度实现亮度更高、更稳定的非线性相干光源,人们充分利用表面等离激元共振和高介电常数的米共振的电磁场局域增强效应来提高非线性频率转换的效率。利用局域表面等离激元共振可以将电磁场局域到亚波长量级空间的能力,能够极大地提高二次谐波[10-25]、三次谐波[26-28]、四波混频[29-32]、高次谐波[33-37]等非线性过程的产生效率,为此大家设计了金属尖端[15]、蝴蝶结(bowtie)[27, 37]等结构,在很小的间隙里面实现极大的电磁场局域增强,并且提出了宽带共振结构[20]、双共振结构[11, 21],在基波处和谐波产生处均引入共振,提高非线性的频率转换效率。进一步地,在双共振的基础上,可以利用法诺共振降低金属微纳结构在基波处的远场辐射损耗,增大其在谐波产生处的远场辐射损耗,使得其在基波处电磁场强度更高,并且产生的谐波更多地以辐射的形式消耗掉,提高谐波的产生效率[38]。此外,除了利用金属微纳结构内禀的非线性响应,还可以将金属微纳结构与高非线性极化率材料结合起来[39-50]。例如,通过设计钛酸钡/金球壳结构,将电磁场局域在钛酸钡材料体内,充分结合钛酸钡材料的高二阶非线性极化率与金球壳结构的电磁场局域增强效应这两个优点,实验中复合结构测量的二次谐波相比于没有金球壳时增强了500倍[41]。
以上工作都是基于单个金属微纳结构增强非线性频率转换过程。另一方面,金属超构表面即金属微纳结构阵列可以提供更多的自由度增强非线性频率转换过程,例如超构表面基元结构的对称性、相互取向、距离等等。大多数超构表面的组成材料是多晶态的,因不满足对称性破缺要求,其产生二次谐波的效率很低。为了解决这一问题,研究者们设计了很多非对称性结构产生二次谐波,例如L型[11, 51-52]、T型[19]、G型[53-54]、U型[12, 16, 55-57]、纳米杯(nanocup)[22]等等,其中Mattias等人首次设计了U型环超构表面实现了二次谐波的增强[57],值得注意的是,满足对称性破缺条件是相对于基波的偏振方向的,如图1(a)所示,当基波偏振方向平行于U型环底部时,可以激发出电共振、磁共振以及二次谐波,二次谐波的偏振方向和基波的偏振方向相互垂直;当基波偏振方向垂直于U型环底部时,只能够激发出电共振,二次谐波信号很弱。这是因为当基波偏振方向平行于U型环底部时,对称性破缺条件满足,其垂直于U型环底部时,对称性破缺条件不满足。此外,可以通过调控超构表面的排布增强谐波的产生。如图1(b)所示,当超构表面基元结构相互之间距离小于基波波长时,基元结构相互之间距离越近,耦合作用越强,损耗越大,线性谱上表现为谱线变宽,基元结构相互之间距离越远,稀释效应越凸显,因此存在一个临界距离使得二次谐波的强度最大[55]。如图1(c)所示,当超构表面基元结构相互之间距离和基波波长相当时,线性瑞利异常模式与基元结构共振模式相互作用产生线性表面晶格共振模式,稀疏的超构表面能够获得比稠密的超构表面强五倍的谐波信号[52]。此外,线性领域的瑞利异常可以推广到非线性领域[56],以二次谐波为例,满足关系式
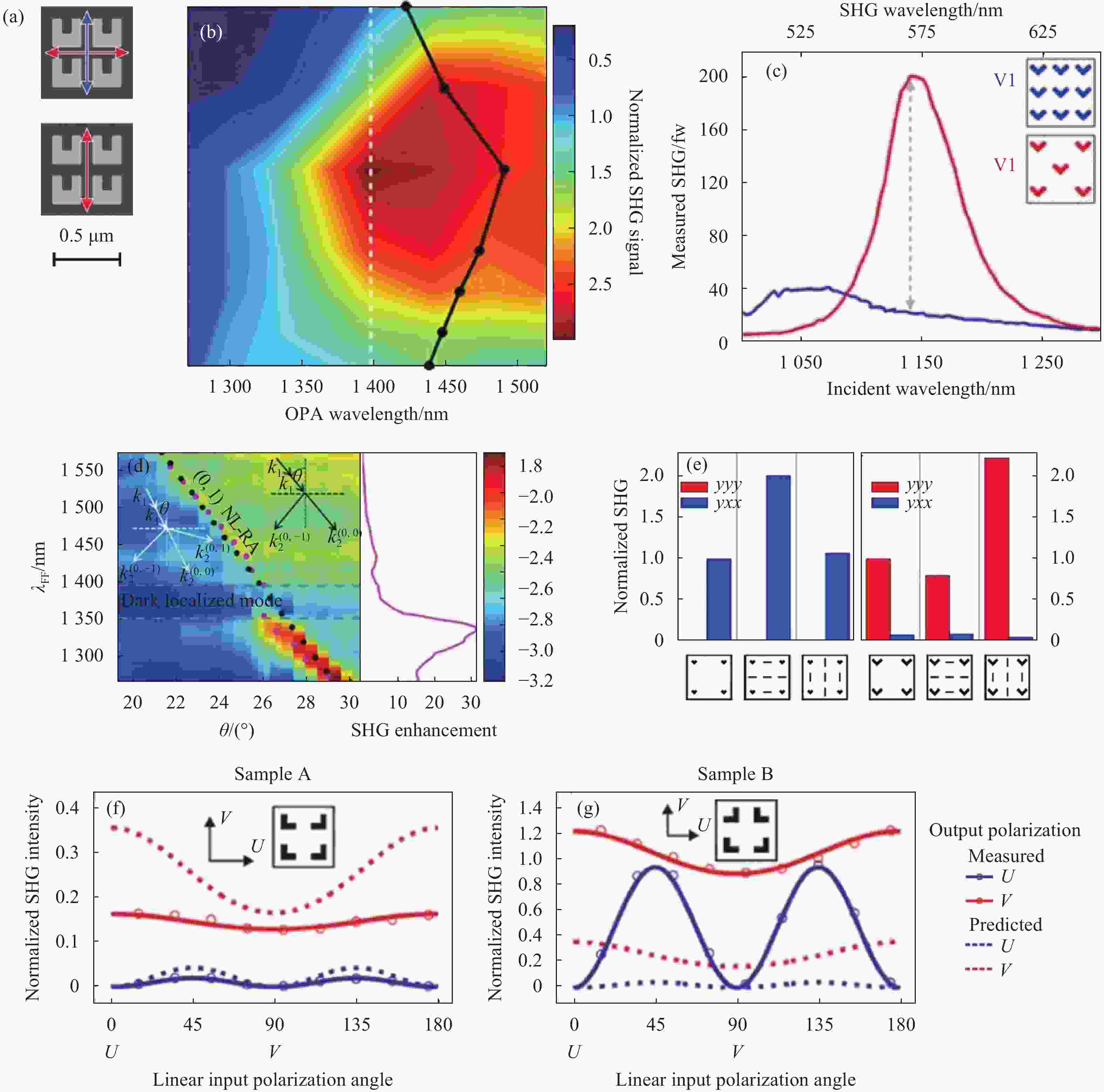
Figure 1. Nonlinear enhancement based on plasmonic metasurface. (a) U-ring resonators magnetic resonance enhances the generation of second harmonics; (b) Relationship between signal strength of the second harmonic wave and inter-distance of U-ring resonators on the metasurface; (c) When linear surface lattice resonance exists on the metasurface of L-ring resonator, the sparse metasurface gets a second harmonic signal 5 times stronger than the dense metasurface; (d) Due to the existence of nonlinear surface lattice resonance, a signal 30 times stronger than the normal incidence of the fundamental wave is obtained by the oblique incidence of the fundamental wave on the U-ring metasurface; (e) Influence of the mutual orientation of metasurface of L-ring resonator on the second harmonic generation; (f) Asymmetric structures are arranged near symmetrical structures to enhance the generation of second harmonics
即可实现二次谐波对应的非线性瑞利异常,其中
${{\mathop {k}\limits^{\rightharpoonup} }_{{\rm{FW}}}}$ 、${{\mathop {k}\limits^{\rightharpoonup}} _{{\rm{S}}}}$ 、${{\mathop {G}\limits^{\rightharpoonup}} _{{m_1},{m_2}}}$ 分别是基波波矢、表面波波矢以及周期性结构提供的倒格矢,m1、m2为整数。如图1(c)所示,当非线性瑞利异常模式与基元结构共振模式耦合时,产生非线性表面晶格共振模式。以斜角度入射能够获得比正入射强30倍的二次谐波信号,这是因为本身是暗模式的基元结构共振通过非线性瑞利异常模式的集体相互作用变成了亮模式。超构表面基元结构的相互取向也能够对二次谐波产生巨大的影响,如图1(d)所示,略微改变超构表面基元结构的相互取向,二次谐波强度可以变化50倍[58]。更有意思的是,虽然对称性结构产生二次谐波效率低,但是在非对称性结构附近排布对称性结构能够影响整个超构表面的电磁场模式,如图1(e)所示,提高其在基波处的光密度,进而增强二次谐波的产生[51]。相对于金属结构而言,一方面,介质结构具有高损伤阈值、低损耗的优点。另一方面,表面等离激元的电磁场主要分布在金属微纳结构与周围环境介质的界面处,体非线性极化率没有得到充分的利用,而介质微纳结构的电场主要分布在介质材料内部,可以避免这一问题。基于这两个优点,研究者们设计了大量介质结构,利用其电共振、磁共振来增强二次谐波[59-64]、三次谐波[65-70]的产生。相对于二次谐波而言,三次谐波对于对称性的要求低,可以充分利用介质微纳结构的体三阶非线性极化率,使得其在相较于金属微纳结构电磁场局域增强效应弱的情况下,实现更高的三次谐波转化效率。如果采用非对称型组成材料,例如砷化镓铝[60-61]、砷化镓[64, 68]、单晶硅[59]、氧化锌[62]等等,满足对称性破缺要求,体二阶非线性极化率和表面二阶非线性极化率均得到有效利用,依然可以实现二次谐波的增强。如图2(b)所示,借助基于砷化镓的超构表面的三个优点:材料本身的高非线性极化率、基元结构的电磁场局域增强效应、降低的相位匹配要求,其上可以同时发生11种频率转换过程[68]。

Figure 2. Nonlinear enhancement of all-dielectric metasurfaces. (a) A metasurface consisting of a disk and a rectangular bar is used to enhance the generation of third harmonics by fano resonance;(b) GaAs based all-dielectric metasurface can simultaneously undergo 11 frequency conversion processes; (c) All-dielectric metasurface uses anapole mode to achieve third harmonic generation enhancement and generates 185 nm vacuum ultraviolet light; (d)
$9 \times 9$ metasurface of silicon particles, in which the symmetry of silicon particles in the x direction is broken, and the symmetry in the y direction is maintained. The quasi-BIC model is used to enhance the third harmonic by five orders of magnitude; (e) Two different zones are designed on the metasurface of silicon particles: shrinkage and expansion of the hexamer distribution. The third harmonic nonlinear characterization of bulk and edge states can be realized by changing the fundamental wavelength除了采用超构表面基元结构的电共振、磁共振来增强非线性频率转换过程,还可以通过调节其不同模式的相互作用,实现更好的电磁场局域增强以及更高的Q值。文中主要介绍三类:anapole模式[67, 71-74]、法诺共振模式[69-70]、BIC模式[75-77]。通过调节基元结构的尺寸,使得其电偶极子和环形偶极子的远场辐射发生干涉相消,可以获得anapole模式,该模式可以极大地减少辐射损耗,使得电磁场能量更多地局域在介质颗粒的内部[78]。如图2(c)所示,通过调节二氧化钛圆柱纳米颗粒的直径可以在基波处获得anapole模式,在共振位置处,该结构的透射极大地降低,产生了185 nm的紫外三次谐波,相对于同样厚度的无图案薄膜,三次谐波获得了180倍的增强[67]。此外,法诺共振因其线宽窄、
Q值高的特点也被应用于谐波产生的增强,法诺共振由一个亮模式和一个暗模式相互作用得到,在正入射条件下,亮模式可以被直接激发但暗模式不能,需要通过亮模式间接激发暗模式,两种模式相互作用产生法诺共振。如图2(a)所示,通过设计由介质圆盘和介质矩形棒组成的超构表面,直接激发介质矩形棒的共振(亮模式),间接激发介质圆盘的共振(暗模式),两种模式相互作用产生法诺共振,使得三次谐波的产生获得了五个数量级的增强[70]。BIC模式可以看成是一种特殊的法诺共振,关于BIC模式的详细介绍可以参阅参考文献[79]。尽管获得BIC模式需要无限大的结构、无限大的或者0磁导率,在实际中不能实现,但是可以参照BIC模式的物理机制设计出高Q 值模式,这样的模式称为准BIC模式[80-81]。例如,通过调节圆柱纳米颗粒的直径,可以使得该结构的径向共振和轴向共振发生耦合,在远场辐射发生干涉相消,实现准BIC模式,Q值高达 $188 \pm 5$ ,实现了二次谐波的极大增强[75]。BIC模式还可以通过打破对称性得到,如图2(d)所示,在基于硅的$9 \times 9$ 超构表面中,打破基元结构$x$ 方向的对称性,$y$ 方向保留对称性,在保证准BIC模式能够被激发的同时,获得了很高的Q值( $ \sim{10}^{4} $ )。使得三次谐波获得了五个数量级的增强,并且反常地观测到了二次谐波的产生[77]。近年来,研究者们开始研究超构表面的非线性拓扑性质,在拓扑非平庸“之”字型硅颗粒超构表面中,利用拓扑磁共振边界态的局域场增强效应和鲁棒性这两个优点,实现了三次谐波的稳定增强,此外还可以通过单个硅颗粒的磁共振与超构表面的拓扑电共振边界态相互作用,改变基波波长或者入射方向控制三次谐波的产生热点[82]。进一步地,在超构表面中设计两种不同的区域;收缩和扩张的六聚体分布,如图2(e)所示,在两种区域的边界上存在拓扑保护螺旋边界态,改变入射基波波长,可以实现体态、边界态的三次谐波非线性表征,并且边界态的传播方向取决于三次谐波的偏振态[83]。级数五次以上的谐波称为高次谐波,可以被用来制备超短极紫外光源,因其转化效率很低,需要激光脉冲强度超过
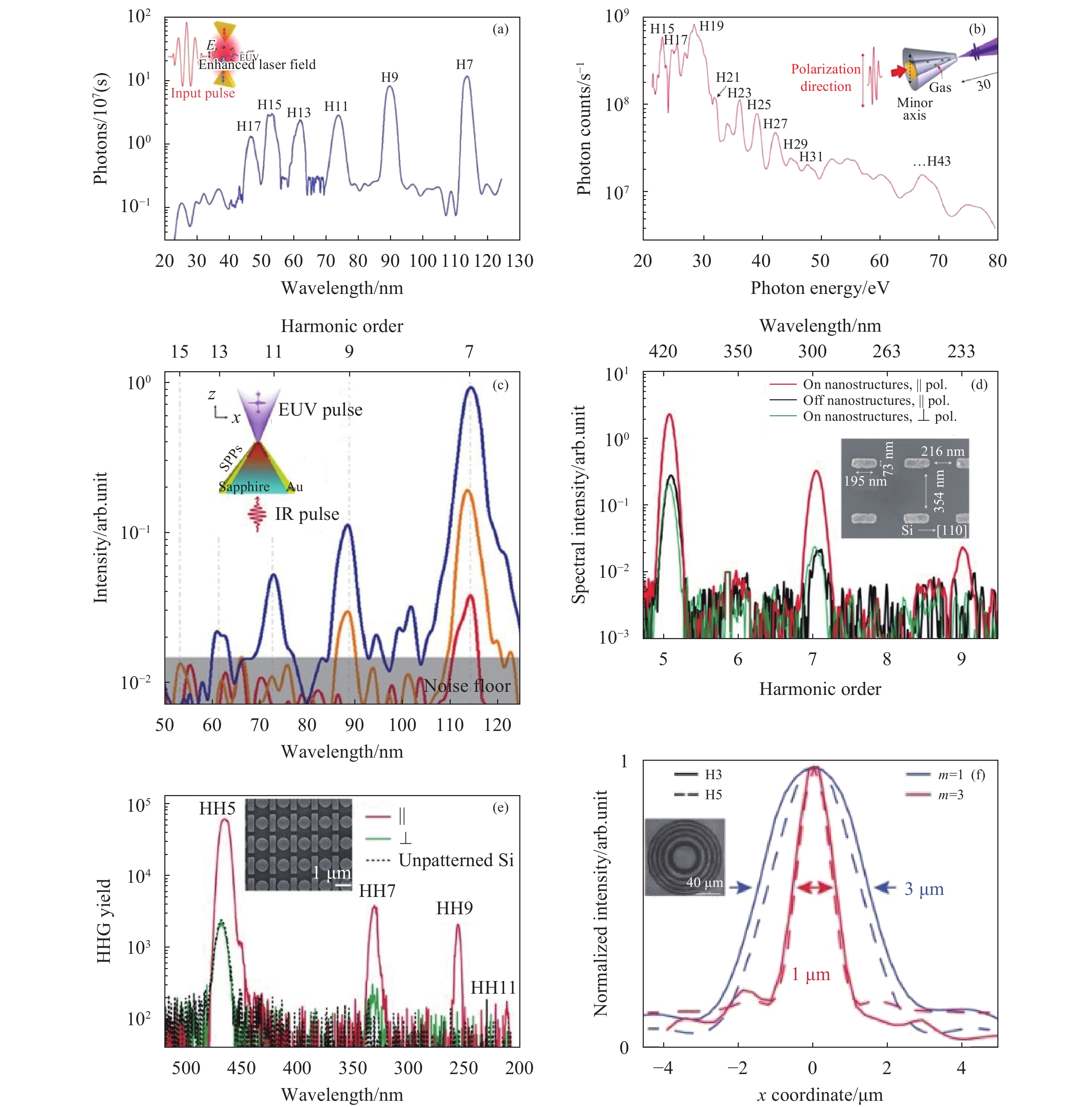
${10^{13}}\;{\rm{W}}/{\rm{c}}{{\rm{m}}^2}$ 。为了解决这一问题,早期的研究者们将表面等离激元的电磁场局域增强效应和稀有气体结合起来用于高次谐波的增强,如图3(a)所示,将激光照射在通有氩气的蝴蝶结阵列结构上,借助蝴蝶结结构间隙的极大电磁场局域增强效应可以实现高次谐波的增强[37]。如图3(b)所示,利用锥形波导表面等离极化激元的电磁场局域增强效应增强稀有气体的高次谐波的产生[36]。由于气体密度低,在电磁场局域增强位置的分子数目少,电磁场局域增强效应没有得到有效利用,全固态增强高次谐波体系可以解决这一问题。如图3(c)所示,将稀有气体更换为单晶的蓝宝石尖端,利用锥形金属波导的电磁场局域增强效应增强了波长为60 nm高次谐波[34]。进一步地,通过在单晶硅衬底上制备金纳米棒超构表面,如图3(d)所示,使得高次谐波的强度比没有金纳米超构表面时强10倍[33]。然而,基于表面等离激元增强高次谐波的体系依然面临着损伤阈值低、加工困难的问题,全介质的超构表面可以很好的规避这一困难。在蓝宝石衬底上镀上一层225 nm的单晶硅膜,制备的超构表面基元结构如图3(e)所示,相对于平整单晶硅膜而言,利用此结构的法诺共振可以使高次谐波获得两个数量级的增强[84]。此外,微纳结构不仅可以被用于增强高次谐波的产生,还可以被用于调控高次谐波的远场辐射,通过向单晶硅膜注入镓离子,影响硅材料的电子能级,制备出菲涅尔波带片,如图3(f)所示,成功将产生的高次谐波聚焦成衍射极限大小的亮斑[85]。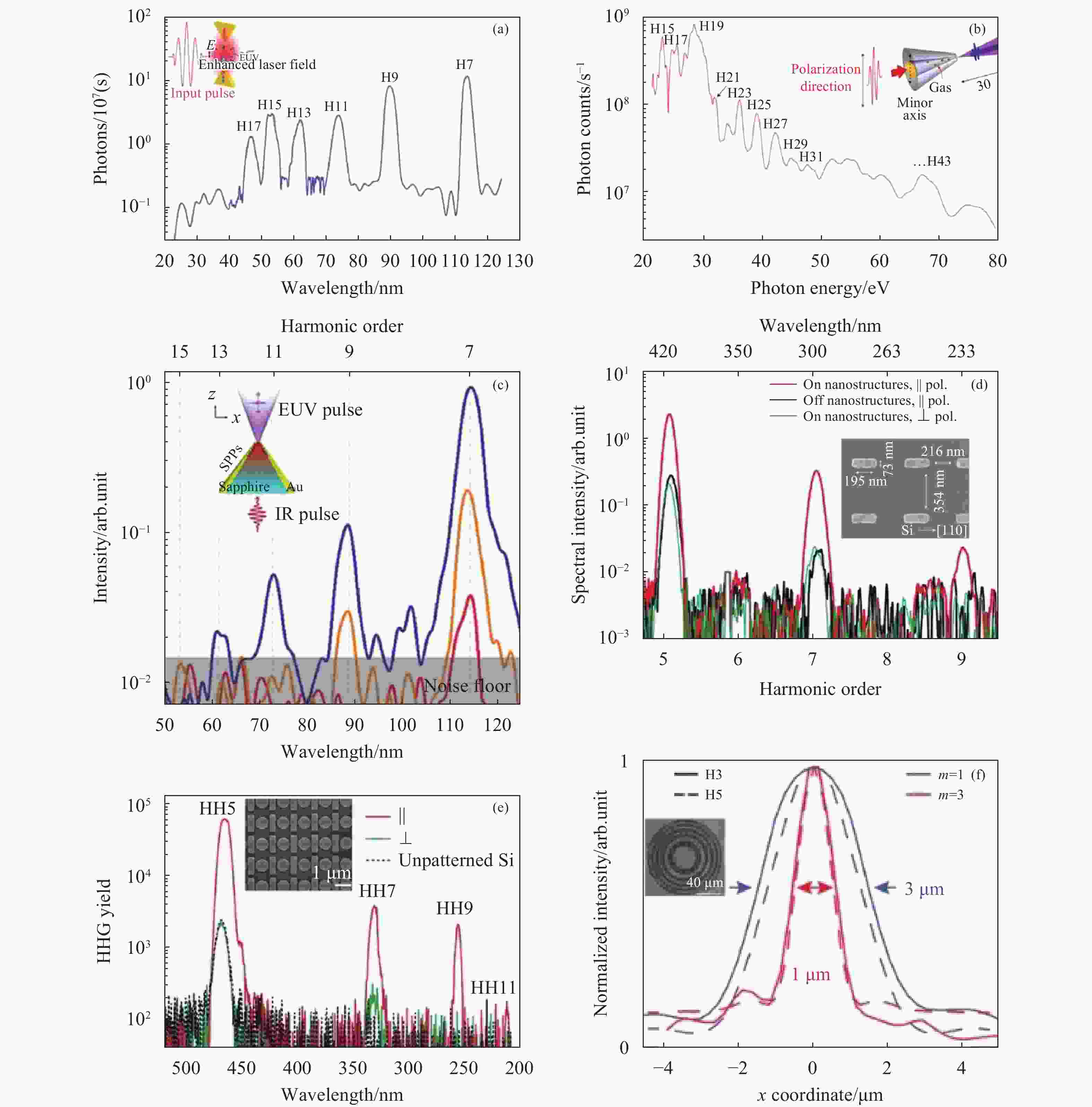
Figure 3. Resonance enhancement and control of high harmonics. (a) Generation of high order harmonics is enhanced by the use of local surface plasmon resonance of the Bowtie structure. (b) Generation of high harmonics is enhanced by using surface plasmon resonance of three-dimensional waveguide structures; (c) Use of conical metal waveguides and sapphire in solid systems to enhance the generation of high harmonics; (d) Preparation of gold nanoparticle arrays on monocrystalline silicon films; (e) Etching silicon film on sapphire substrate and introducing fano resonance to enhance high harmonics; (f) Focus of the 3rd and 5th harmonics is achieved through the preparation of Fresnel zone plate by gallium ion implantation into the silicon film
-
上一节中论述了基于超构表面的非线性增强效应,接下来主要介绍当入射光为圆偏振态时,谐波的产生与对称性的关系。对于超构表面而言,对称性从两个方面去考虑:组成材料的微观对称性与基元结构的宏观对称性,正如上文中所提到的,通过设计对称性破缺的结构,能够在多晶态的微纳结构上观测到二次谐波的产生。这里大多数是考虑线偏振光入射时,中心对称性对于二次谐波产生的影响。此外,当入射光为圆偏振时,由于自旋轨道耦合作用,将超构表面基元结构旋转一定的角度会给入射光以及非线性电偶极子引入几何相位。特别地,对于一个
$n$ 重对称性的结构,为了保持基元结构的非线性极化率张量在旋转角度$2\pi /n$ 时保持不变,必须满足关系式$m = nl \pm 1$ ,即选择性定则,其中$m,n$ 分别为谐波产生的级数和基元结构的对称性,$l$ 为整数,正、负号分别对应产生的谐波与基波偏振方向相同、相反的情况[86]。在二次谐波的产生过程中,使用右旋偏振光激发三种不同对称性重数的结构,当$m = 2,n = 1$ 时,产生的二次谐波中包含左旋和右旋两种成分;当$m = 2,n = 3$ 时,只能产生与基波的偏振方向相反的二次谐波;当$m = 2,n = 6$ 时,上述关系式不能得到满足,因此二次谐波产生的过程被禁止[87]。基于同样的原理也可以讨论对称性对三次谐波的产生过程的影响,当$m = 3,n = 2$ 时,产生的三次谐波中包含左旋和右旋两种成分;当$m = 3,n = 3$ 时,不能满足上述关系式,因而三次谐波产生的过程被禁止;当$m = 3, n = 4$ 时,产生的三次谐波的偏振方向与基波偏振方向相反[86]。研究非线性频率转换与对称性的关系对于设计超构表面具有普适的指导意义。除了超构表面基元结构的多重对称性对于谐波产生级数的限制,超构表面的谐波产生效率也依赖于入射基波的偏振态。近年来,非线性手性光学技术已经被成功应用于表征化学表面、生物分子的手性特征等相关方面。相对于三维超构材料而言,超构表面不仅可以降低加工难度,而且可以很好与生物分子的物理界面相兼容。在线性光学领域里,使用圆二色性和光学活性来衡量手性物质对于左旋和右旋圆偏振光之间相位和吸收的差异。仿照线性光学领域里的圆二色性,不同圆偏振态的基波产生谐波的效率差异可以用非线性圆二色性来衡量,例如二次谐波的圆二色性定义为:
式中:
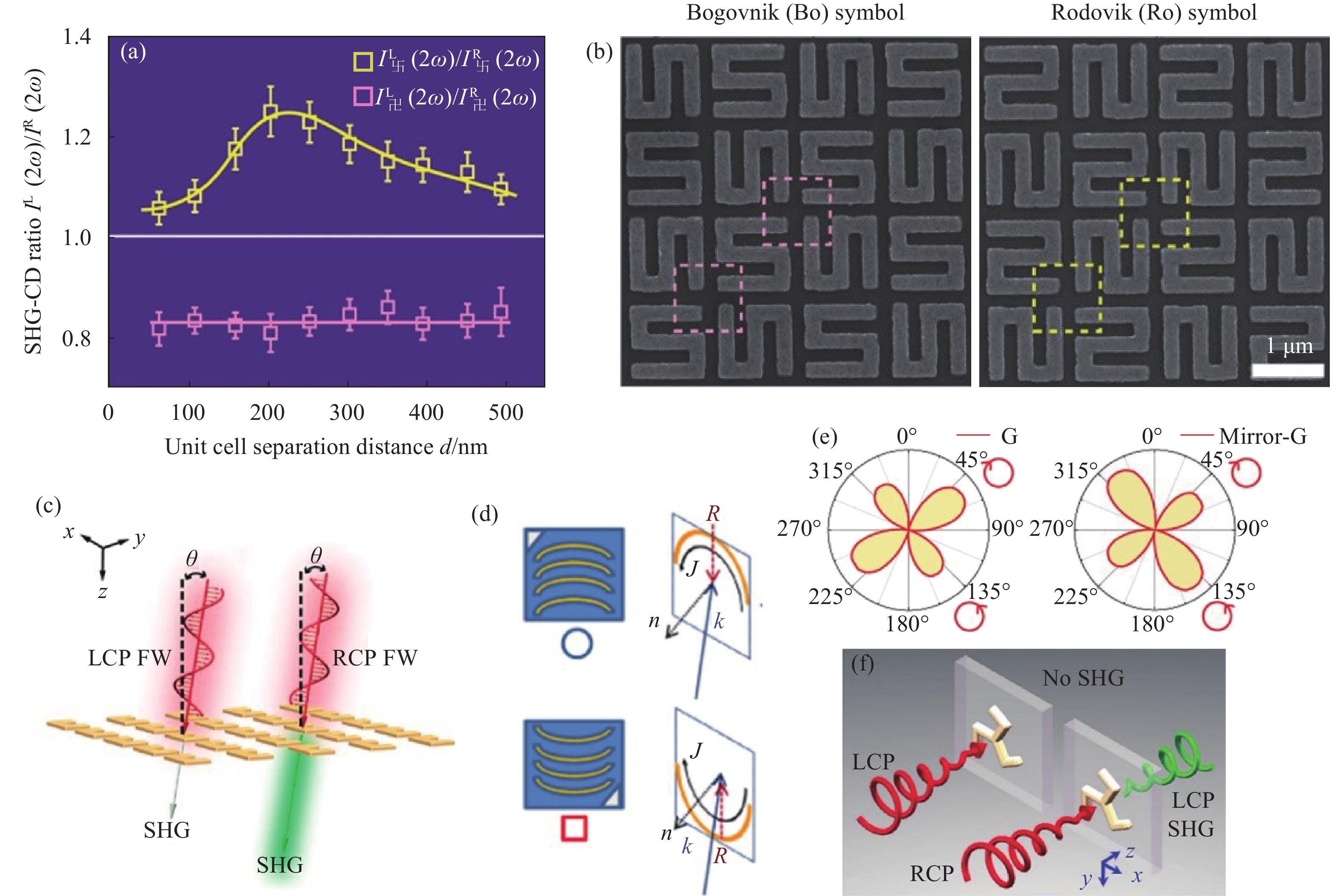
$I_{\rm{L}}^{2\omega }$ 、$I_{\rm{R}}^{2\omega }$ 分别代表产生的左旋、右旋偏振基波入射时二次谐波的信号强度,其他级次谐波的圆二色性定义依此类推。事实上,研究者们已经通过调节超构表面的基元结构排布、对称性、周期以及基波入射角度来研究非线性手性响应过程。如图4(a)所示,在G型超构表面的特定排布下,左、右旋偏振态基波产生的二次谐波效率存在差异,均匀排布的G型超构表面则没有这种现象[54]。如图4(b)所示,调节超构表面基元结构的距离,进而调控基元结构之间的耦合作用,也可以实现二次谐波的圆二色性,其值为52%[88]。如图4(c)所示,当基元结构具有多重对称性的时候,其谐波的产生不仅满足选择性定则,通过调节不同非线性极化率之间的相位差,还可以实现接近100%的非线性圆二色性[89]。此外,非线性圆二色性并不局限于超构表面的内禀结构手性,通过引入外禀结构手性,在非手性结构中其也可以被观察到[90-91]。例如,如图4(d)所示,当基波以斜角度入射到弯曲金纳米线超构表面时,二次谐波圆二色性随基波入射角度增大而增大,最高为50%[90]。进一步地,为了能在小角度入射条件下获得高的非线性圆二色性,如图4(e)所示,在基波斜角度入射的条件下,当超构表面基元结构的周期和基波的波长相当时,表面晶格共振的存在增强二次谐波的圆二色性,实验中测量到在基波以$ \pm {10^\circ }$ 小角度入射条件下,二次谐波的圆二色性最高可为60%[91]。此外,还可以利用等离激元超构表面增强非线性光学活性,例如,由于电磁场局域增强效应,U型环超构表面上观察到的非线性光学活性比碘酸锂晶体强$3 \times {10^7}$ 倍[92]。研究基于超构表面的非线性手性响应过程对于设计出灵敏度更高、体积更小的化学表面及生物分子表征器件具有重要的指导意义。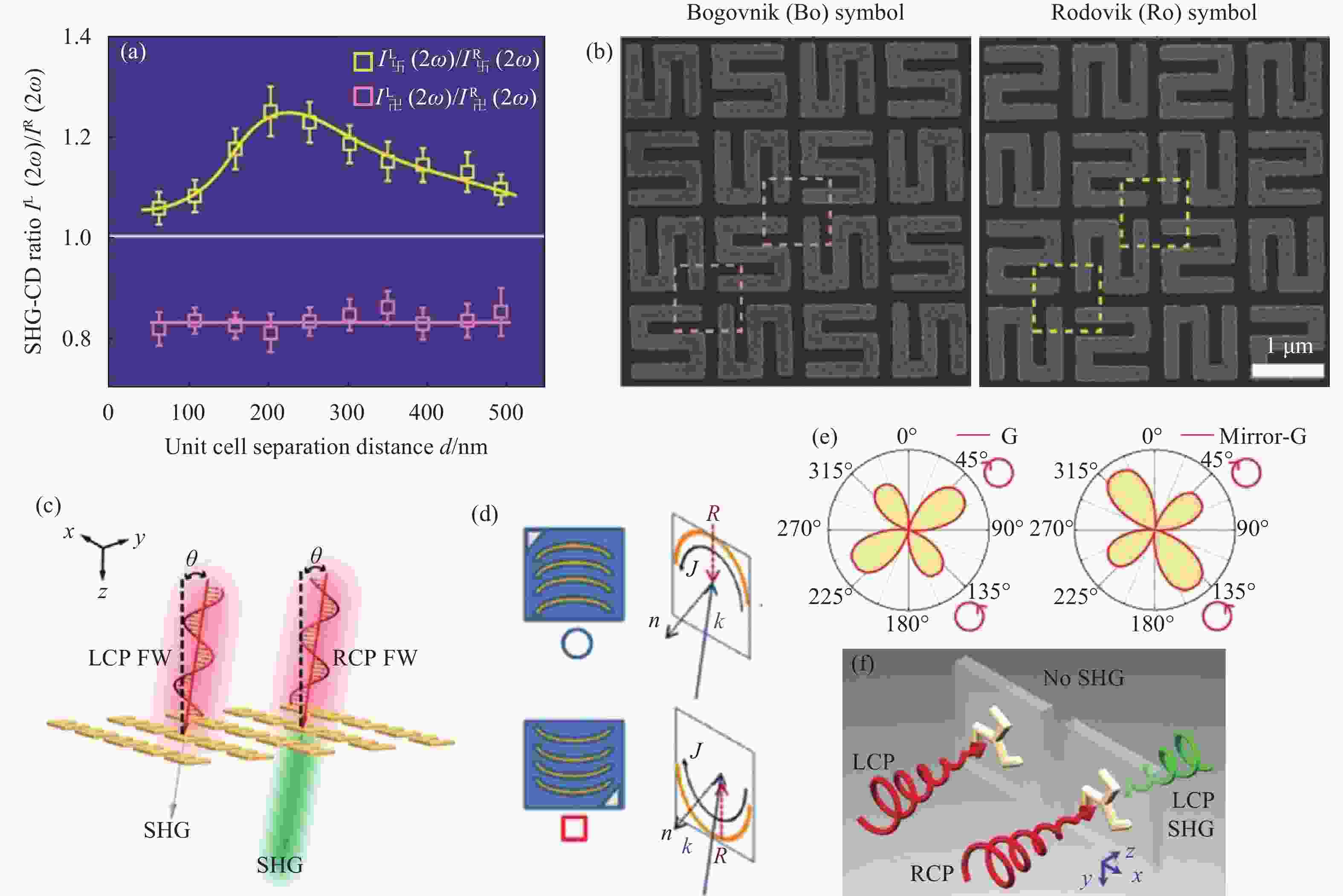
Figure 4. Relation between the harmonic generation and symmetry. (a) Nonlinear circular dichroism of metasurface of G-type element in particular configuration; (b) Nonlinear circular dichroism of S-type element metasurface; (c) Second harmonic and third harmonic nonlinear circular dichroism of the chiral structure with triple and quadruple symmetries respectively; (d) Introducing extrinsic structure chirality by the oblique incidence of fundamental wave on the bent gold nanowire array; (e) Presence of surface lattice resonance enhances the nonlinear circular dichroism on the metasurface of U-ring
-
超构表面不仅能够增强非线性的响应过程,而且可以在亚波长尺度改变局部的非线性相位响应和振幅响应,从而控制其非线性的响应过程。这种方法无缝结合了谐波的产生和操纵,有利于非线性纳米光学元件的小型化和集成化。类似于线性光学领域,基于超构表面的几何相位和共振相位可以实现对非线性谐波产生的相位、偏振调控。超构表面的非线性相位调控是受几何相位概念启发的。如图5(a)所示,当把U型环基元结构翻转时,其二次谐波响应引入一个
$\pi $ 相位,Segal等人设计的二元相位分布的开口环阵列实现了对二次谐波发射方向的操控[93],基于此设计,Wolf等人提出了相控阵列光源的概念,它可以同时充当分束器和偏振分束器[94]。更进一步,利用几何相位可以实现对非线性谐波产生的连续相位调控,可以实现对谐波任意角度的偏振操作[95]。如图5(b)所示,Li等人将纳米基元结构相对于实验室坐标系旋转角度θ以引入几何相位,则n阶非线性极化率可以表示为${\alpha _ \pm }\sim \exp{{ i}}\sigma \left( {n \pm 1} \right)\theta$ ,其中正负号下标分别代表谐波与基波圆偏振态相同或相反的情况,σ = ±1分别代表左、右旋圆偏振态[96]。除了旋转基元结构的方法外,还可以利用超构表面的共振相位来实现非线性响应过程的相位控制。Almeida等人通过改变矩形纳米天线的长宽比也可以实现非线性响应过程的全相位控制[97]。Zang等人将开口环阵列和旋转${90^ \circ }$ 的互补开口环阵列交替排布同时实现了二次谐波产生,圆偏振态的分离,以及四分之一波片的偏振调控[98]。基于超构表面的非线性相位调控技术已被用于实现非线性全息成像,如图5(c)~5(d)所示,相位编码被储存于超构表面的纳米结构中,几何相位的鲁棒性和无色散特性极大地简化了波的操纵。得益于连续的相位控制,非线性超构表面全息术已显示出优于具有离散相位水平的常规深度控制全息术的优势[99-100]。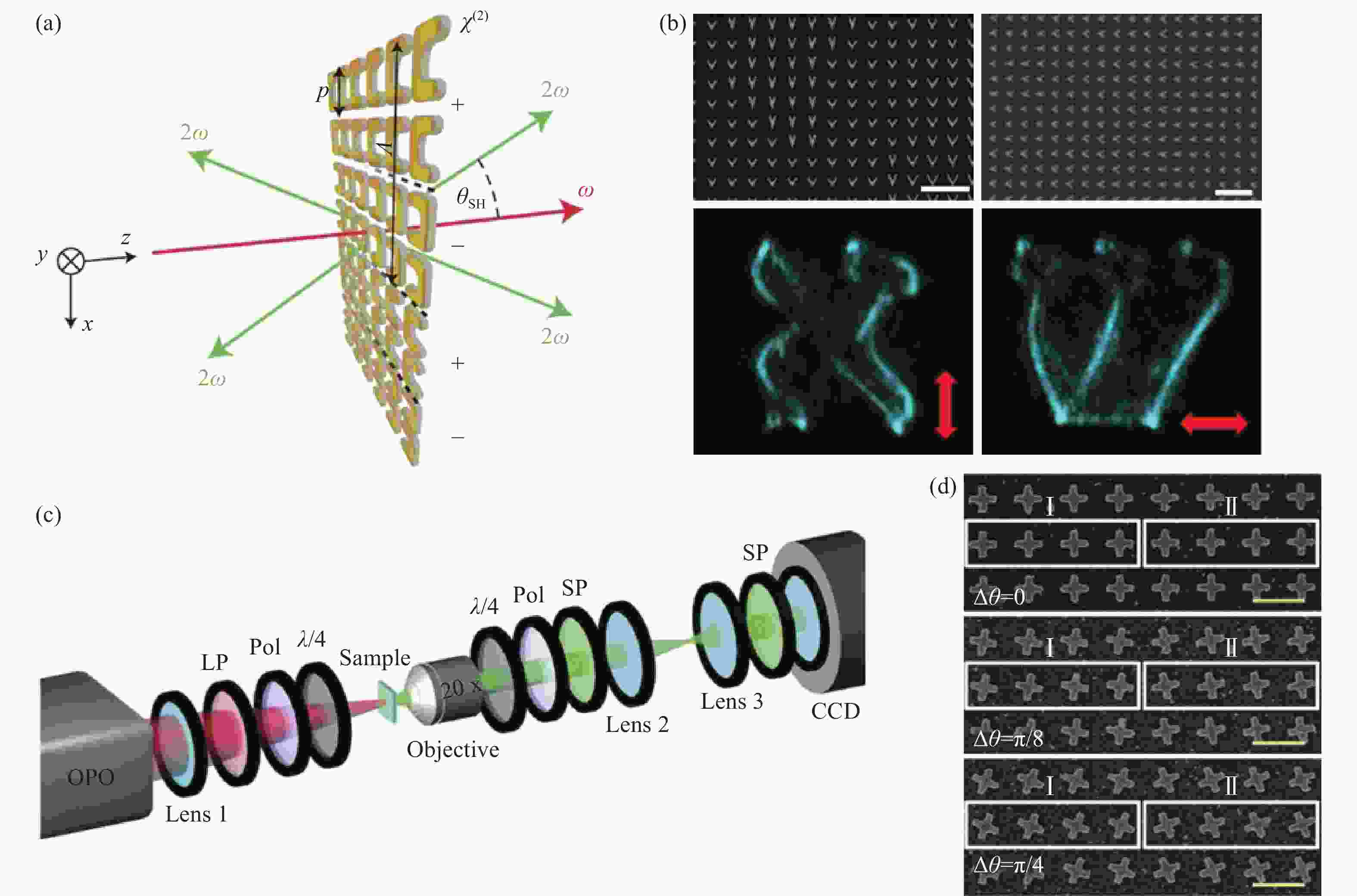
Figure 5. Nonlinear phase control and application based on metasurface. (a) Direction of nonlinear emission is controlled through nonlinear photonic crystals (NPC); (b) Nonlinear phase grating was used for experimental verification of nonlinear phase; (c) Spin and wavelength-dependent holography; (d) Nonlinear metamaterial holography
-
超构表面除了在经典光学领域具有广泛的应用前景,近年来,基于超构表面的量子光学引起了人们越来越多的兴趣,接下主要介绍了微纳结构的量子光学特性。当光与金属微纳结构相互作用时,光子可以和金属表面的自由电子耦合形成表面等离激元。由此产生的局域场增强效应已被用于实现异常光学透射(EOT)和提高非线性光学效率[101]。尽管表面等离激元具有不可避免的能量损耗,但是其独特的光学特性大大增加了人们对局域光场的操纵和调控能力。得益于此,表面等离激元的应用很快被扩展到物理学的其他领域,并与量子光学结合。有趣的是,虽然表面等离激元包含了大量电子的运动和相互作用,但在适当条件下会表现出量子行为,如量子纠缠,量子隧穿以及量子自干涉。如图6(a)所示,Altewischer等人首先观察到表面等离激元的量子纠缠现象,实验发现纠缠光子对经过金膜超构表面转换成表面等离激元后能够保留其量子性质[102]。Moreno等人使用量子理论对该现象进行了解释[103]。如图6(b)所示,Fasel等人进一步指出光子−表面等离激元−光子过程保持了能量时间纠缠[104]。Huck等人通过实验证明了表面等离激元传播中连续变量量子态的鲁棒性[105]。如图6(d)和6(e)所示,Tan等人观察到紧密放置的两个表面等离激元共振器的量子隧穿现象[106]。如图6(c)所示,Kolesov等人发现银纳米线耦合单光子源会激发表面等离极化激元,并推断其具有波粒二象性,类似于单光子[107]。Dheur等人使用特制的表面等离激元分束器观察表面等离极化激元的自干涉现象,从而验证了这一推断[108]。
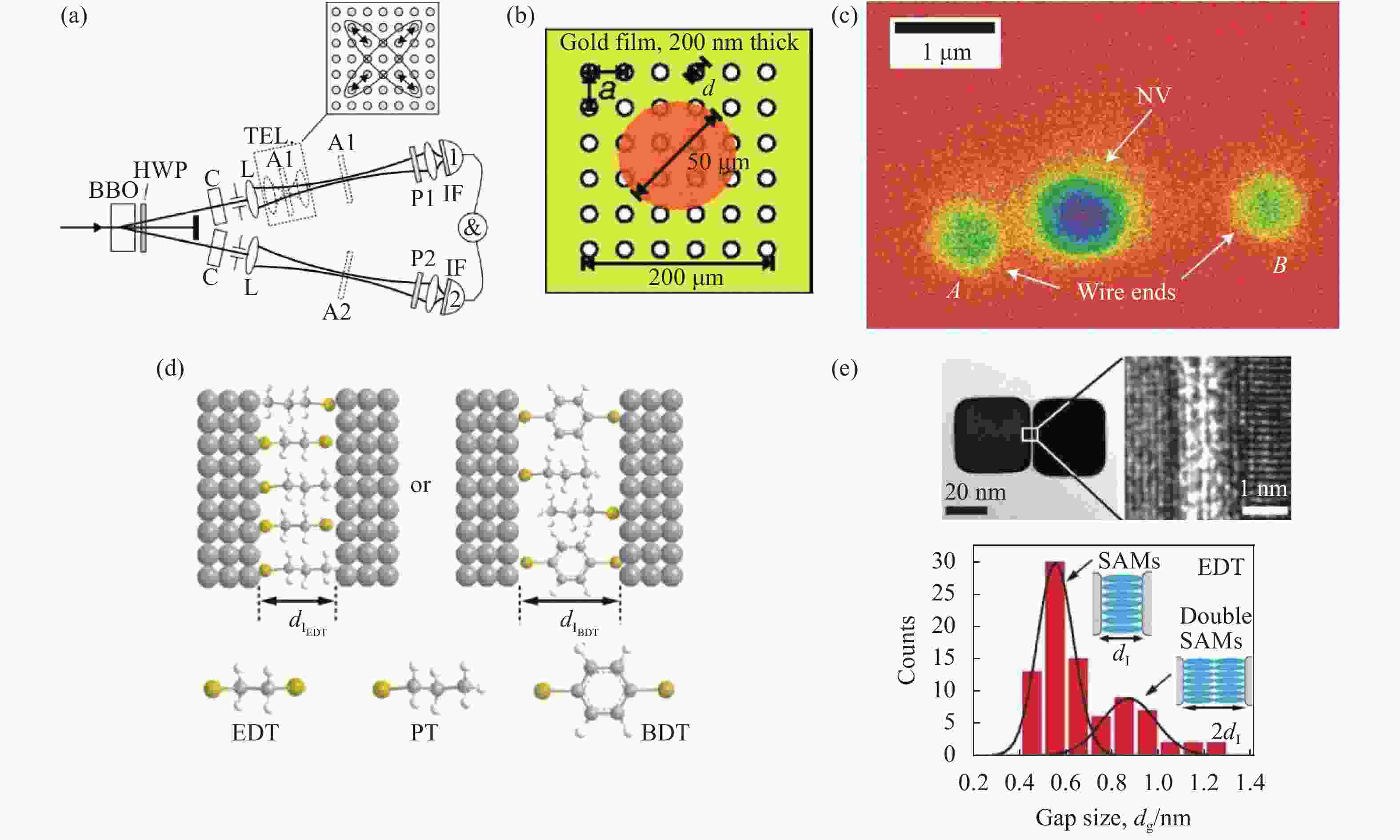
Figure 6. Quantum effects based on surface plasmon. (a) Experimental apparatus and measurement results to verify the true quantum properties of surface plasmon excited separately by entangled photon pairs; (b) Schematic diagram of the experimental apparatus for verifying the energy-time entanglement of the surface plasmon; (c) Sub-poisson statistics of surface plasmon; (d) Schematic diagram of quantum surface plasmon tunnel junction; (e) Direct observation of quantum tunneling between surface plasmon resonators
-
量子纠缠是量子物理区别于经典物理的核心概念之一,其对于量子通信[109-114]、量子计算[115-121]、量子测量及量子传感[122-123]具有重要的意义。为了利用量子纠缠的新奇物理现象来推动量子光学科技的发展,如何获得大面积、稳定、可控以及小尺寸的多光子、高维度纠缠光源成为亟需解决的问题之一。一方面,增大量子纠缠光源的光子数目可以发现更多新奇的量子物理性质。另一方面,在量子通信领域,提高量子纠缠光源的维度能够提供更高的信息容量以及更强的抗干扰能力。此外,在量子计算领域,相比于低维度量子纠缠光源而言,高维度的量子纠缠光源能够提供更高的效率和灵活性。近年来,研究者们利用光子的空间模式、时间−能量、偏振、路径等自由度制备了高维度的量子纠缠光源。该节主要介绍基于超构表面的量子光源的工作。
通过基元结构的材料选择、尺寸调控以及旋转角度等方式,超构材料对于自发参量下转换过程的调节提供了丰富的自由度,因此在制备期望的多光子纠缠态方面具有巨大的应用潜力。目前,产生纠缠光子对最为普遍的方法是利用非线性晶体的自发参量下转换过程。其实,三维超构材料本身也能够发生自发参量下转换过程。当考虑超构材料本身的非线性性质时,Ming等人从理论上证明了通过设计三维超构材料基元结构的尺寸以及排布,可以直接在超构材料上制备期望的量子纠缠态[124]。然而,由于三维超构材料加工的困难以及其基元结构组成材料的低效率自发参量下转换过程,实验上还没有成功制备出基于超构材料内禀非线性响应的量子光源。为了解决这个问题,Li等人创造性地在实验上证明了可以通过将超构表面与非线性晶体结合起来制备高维度、多光子纠缠的量子光源[125]。如图7(a)所示,将超构表面与II型硼酸钡(BBO)晶体粘合在一起,利用超构表面基元结构的光束聚焦功能,将404 nm的激光聚焦在硼酸钡晶体的中心位置,使得在超构表面基元结构的焦点处发生自发参量下转换过程,产生纠缠光子对。当该复合结构产生的纠缠光子对数目为1时,无法区分该纠缠光子对来自于哪一个基元结构,因此可以实现纠缠光子对的路径纠缠,如图7(c)所示,选取三个、四个基元结构,分别表征了三维、四维双光子量子态,保真度分别高达96.6%和95%。此外,还可以通过调节超构表面的相位梯度,调控量子态的产生,如图7(d)所示,其中超构表面相邻基元结构的相位差分别为
$0,{\pi / 2},\pi ,{{3\pi } / 2}$ 。制备的二维双光子纠缠态分别为$\left( {\left| {00} \right\rangle + \left| {11} \right\rangle } \right)/\sqrt 2 $ 、$\left( {\left| {00} \right\rangle + {{i}}\left| {11} \right\rangle } \right)/\sqrt 2$ 、$\left( {\left| {00} \right\rangle - \left| {11} \right\rangle } \right)/\sqrt 2 $ 、$\left( {\left| {00} \right\rangle - {{i}}\left| {11} \right\rangle } \right)/\sqrt 2$ ,保真度平均值高达97.9%。由于该超构表面上产生的纠缠光子对是路径纠缠的,该复合结构可以轻松地实现稳定、紧凑的多光子量子光源,如图7(b)所示,4光子、6光子的符合计数随泵浦功率变化分别满足二次方、三次方关系,充分证明了4光子、6光子纠缠源的成功制备。基于超构表面的量子光源提供了一种稳定、紧凑的高维度、多光子纠缠源,对于推动量子光学技术的发展具有重要的意义。
Figure 7. Quantum light source based on metasurface. (a) Schematic diagram of quantum light source based on metasurface; (b) Characterization of three and four-dimensional two-photon quantum states; (c) Four Bell states were successfully prepared by adjusting the phase gradient of the metasurface; (d) Characterization of multi-photon quantum light source based on metasurface
-
在量子光学领域里面,量子态的调控对于实现量子逻辑门具有举足轻重的作用。常见的光子量子态的调控可以通过线性光学元件实现,例如波片、分束器等。由于超构表面出色的相位调控能力,超构表面可以实现几乎所有线性光学元件的功能,因此超构表面可以被用于光子量子态的调控。如图8(a)所示,利用超构表面的几何相位,使得不同自旋偏振态的光子获得不同的角动量[126],光子经过超构表面的过程表示为:

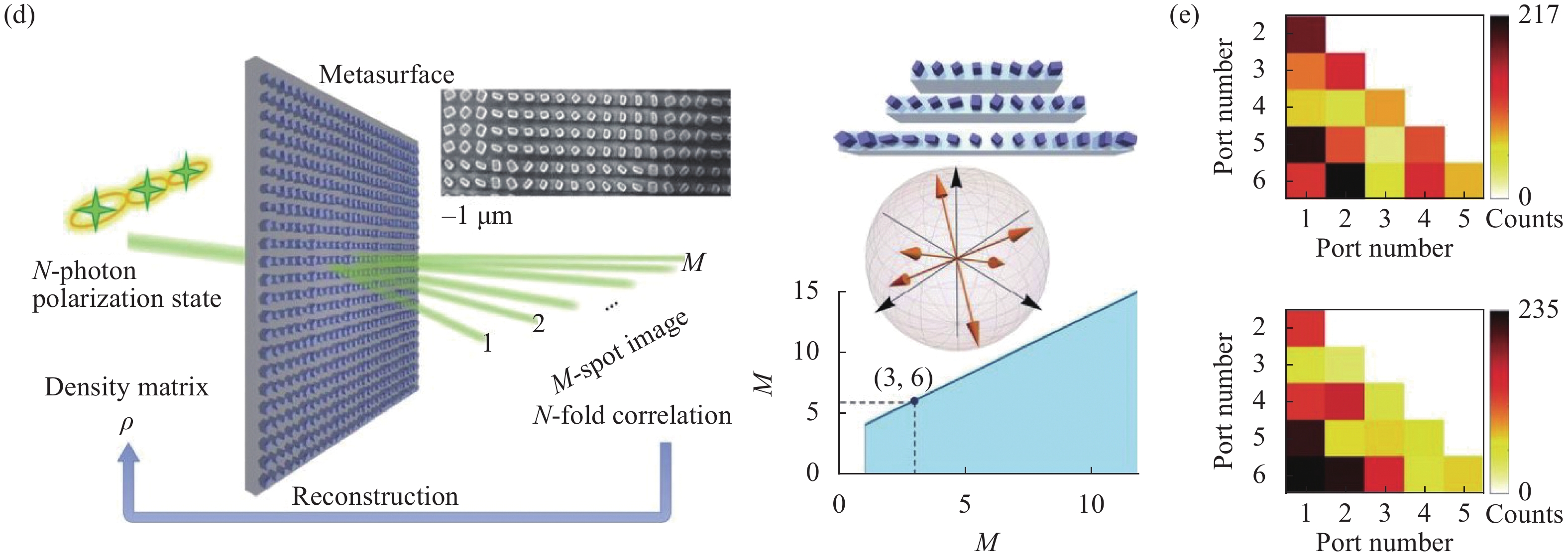
Figure 8. Quantum state manipulation based on metasurface. (a) By using the geometric phase of a metasurface, photons in different spin-polarized states are given different orbital angular momentum; (b) Four Bell states of entanglement between spin and orbital angular momentum of a single photon; (c) Mutual entanglement of spin angular momentum and orbital angular momentum of two photons; (d) On the left is a schematic diagram of quantum state tomography based on the metasurface, and the inset is an SEM image of the metasurface. On the upper right is the nested structure of three different metasurfaces, in the middle is the diagram of the six different polarization states of the metasurface beam splitter, and on the bottom is the relationship between the minimum number of beam splitter and the number of photons; (e) Density matrix of two different two-dimensional two-photon states based on metasurface reconstruction, the fidelity is 95.24% and 98.54%, respectively
式中:
${\sigma _ + }$ 、${\sigma _ - }$ 、$l$ 、$\Delta l$ 分别代表右旋、左旋偏振态、光子携带的轨道角动量以及光子的轨道角动量变化值,其中轨道角动量的变化值取决于超构表面的相位分布。以单光子为例,一个水平偏振、轨道角动量为0的光子的量子态表示为:其中H代表水平偏振。其经过超构表面后,量子态表示为:
实现了单光子自旋角动量与轨道角动量的纠缠。通过设计不同相位分布的超构表面以及对超构表面进行翻转,可以对单光子态进行不同的量子操作,图8(b)为单光子自旋角动量与轨道角动量纠缠的四种贝尔态。基于同样的原理可以获得双光子自旋角动量与轨道角动量的相互纠缠,如图8(c)所示,当纠缠光子对中一个光子的自旋偏振态为
$\left| {{\sigma _ + }} \right\rangle $ 时,另一个光子轨道角动量为1的概率明显大于轨道角动量为−1的情况,反之亦然。此外,还可以利用超构表面对多光子量子态进行投影测量[127],如图8(d)所示,将$ {M / 2}$ 种不同的超构表面嵌套在一起,每一种超构表面都可以实现两种不同椭圆偏振态的分束,该超构表面总共可以实现M种不同椭圆偏振态的分束,$N$ 光子态在超构表面上发生量子干涉,导致$N$ 个光子辐射到不同偏振态通道的行为是相互关联的,通过测量不同组合的偏振态通道,由其符合计数可以反演出$N$ 光子态的密度矩阵。以二维双光子态为例,通过将$三$ 种不同的超构表面嵌套可以获得$六$ 种不同椭圆偏振态的分束,重构二维双光子态密度矩阵至少需要选择15组不同的测量基矢,测量基矢可以通过将超构表面的六个分束通道两两组合获得,图8(e)重构了两种不同二维双光子态的密度矩阵,保真度分别为95.24%和98.54%,这种方法极大地提高了量子态表征的速度与稳定性。相对于介质超构表面而言,虽然金属超构表面具有损耗高的缺点,但是这一缺点可以转化为优点,例如,通过设计吸收具有偏振依赖性的金属超构表面,可以降低非最大纠缠态中特定偏振态的概率,提高量子态的纠缠度,实现对量子态的提纯[128]。此外,还可以利用激光照射在金属超构表面上,控制金属基元结构和衬底的温度,影响超构表面对于光子的响应,实现对量子态的主动调控[129]。 -
基于超构表面还可以实现很多在经典领域不能实现的应用。如图9(a)所示,将金属超构表面的损耗与量子干涉相结合可以增强单个光子或多个光子的全吸收[130-132]。金属超构表面本身对于单光子的吸收是几率性的,但是利用单光子路径自由度的叠加态,使得单光子同时从金属超构表面两端入射,在金属超构表面上发生量子干涉,从而可以实现确定性的单光子全吸收[132]。此外,基于纠缠光子对的非局域相干性,通过测量其中闲置光子的偏振态,可以调控金属超构表面对信号光子的吸收响应[131]。进一步地,金属超构表面可以实现NOON态双光子的高效吸收,效率高达40%,是线性吸收效率的两倍[130]。超构表面也可以应用于量子成像[133],如图9(b)所示,通过在超构表面上压印并叠加两种不同的图案:三角形和五角星,这两种图案分别只能透过两种不同偏振的光。当使用纠缠光子对中的信号光子对该超构表面进行成像时,通过选择性地投影测量闲置光子,可以分别清晰地显示两种图案,并且随着纠缠光子对的纠缠度降低,两种图案的区分度降低。
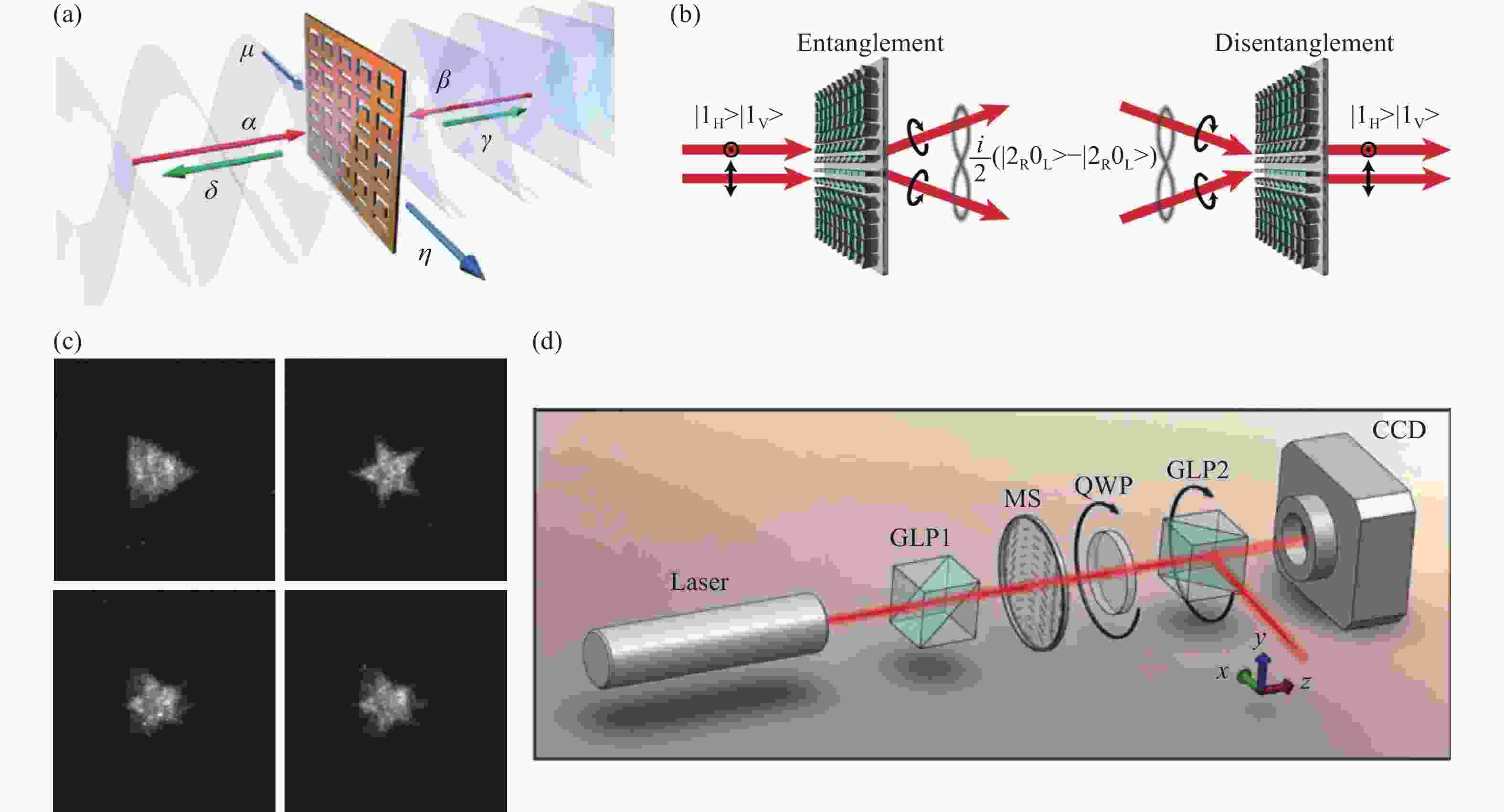
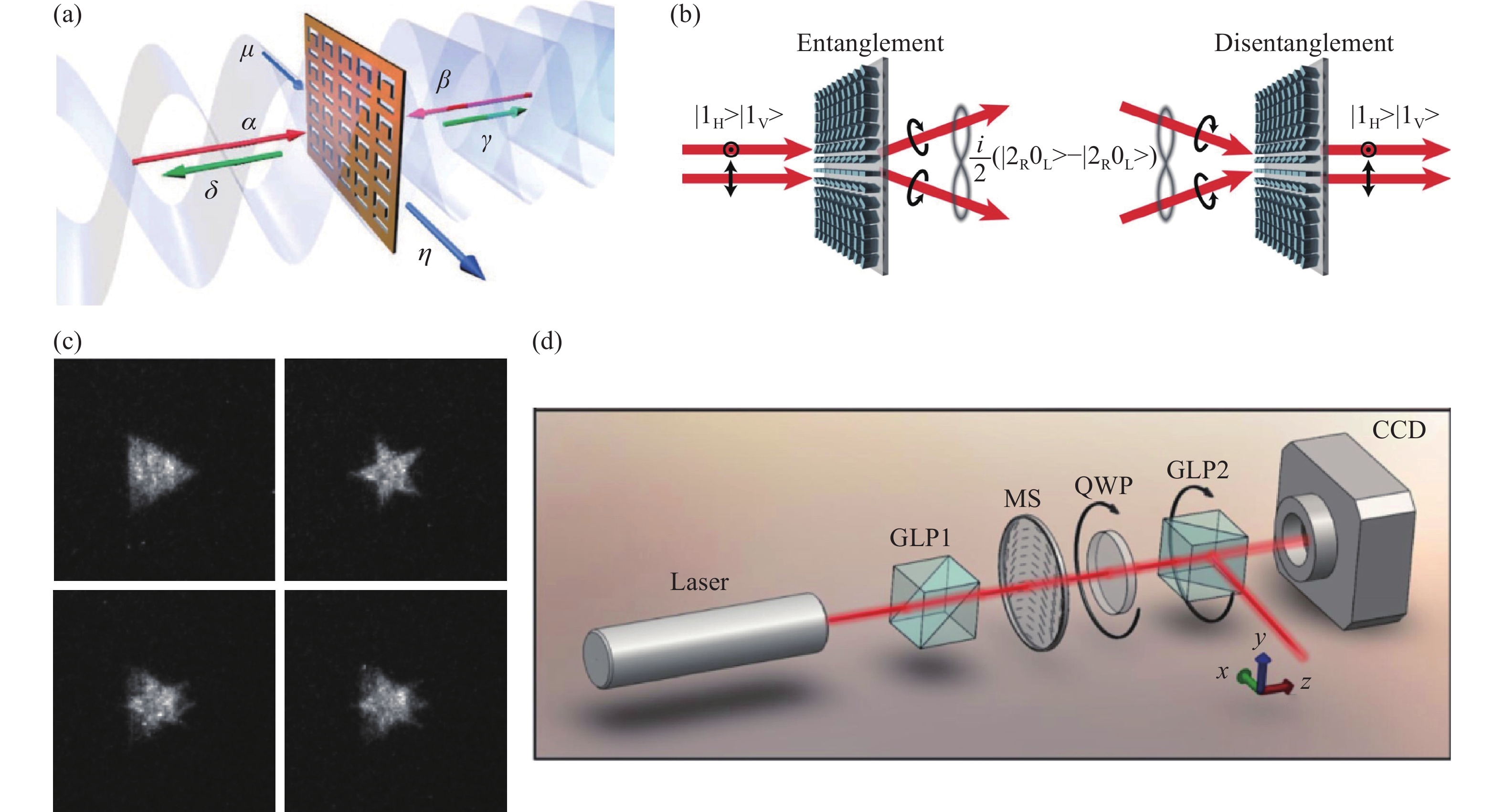
Figure 9. Quantum optical applications based on metasurface. (a) Full absorption of a single photon based on a metal metasurface. The two input channels of the photon α, β, the two output channels of the photon
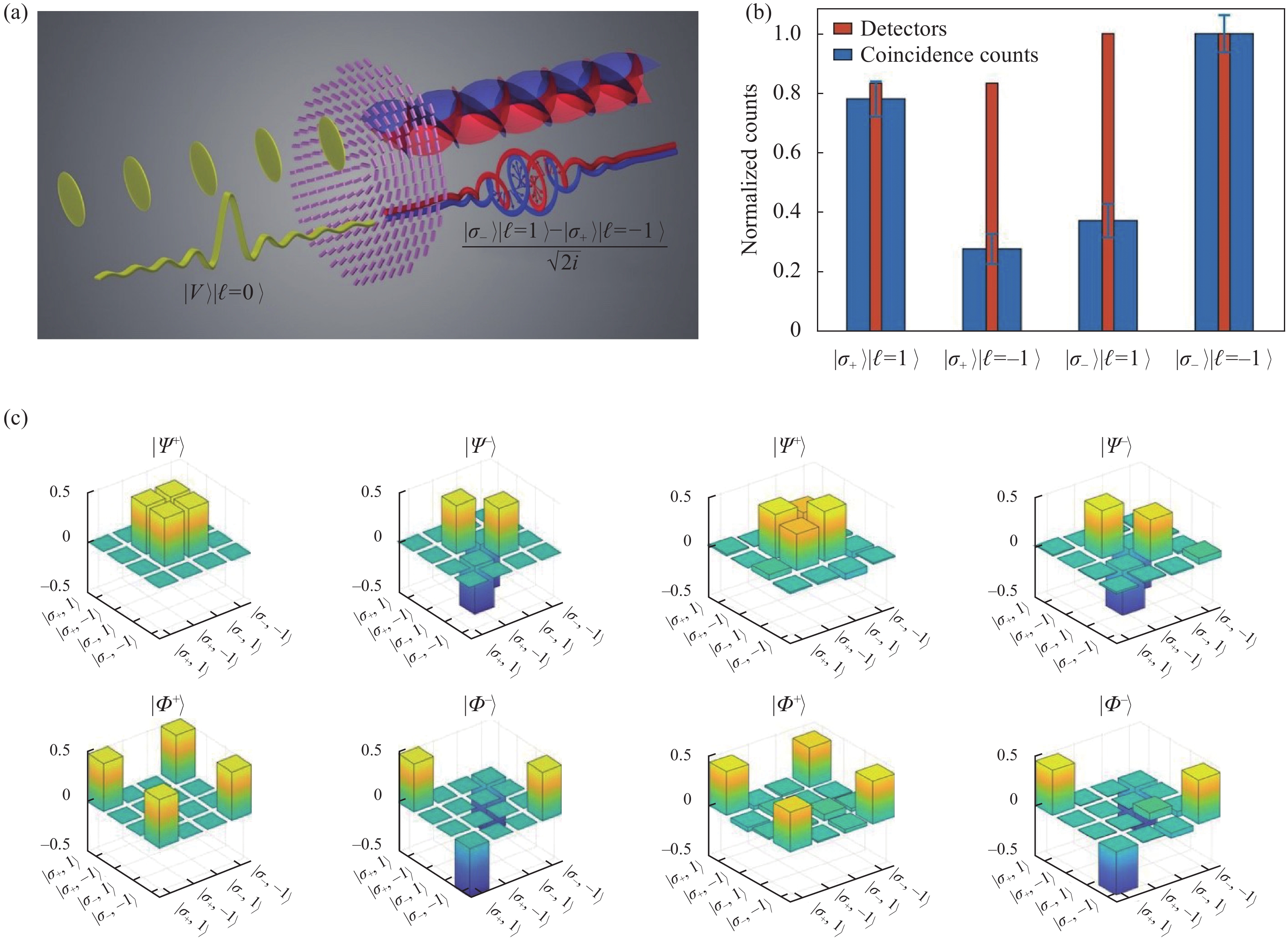
$\delta $ ,$\gamma $ , and the input and output channels of the surface plasmon$\mu $ ,$\eta $ are shown. In the figure below, the normalized count of the photon output channel$\gamma $ ,$\delta $ changes with the position movement of the metasurface, where the photon output channels are normalized relative to the photon input channels$\beta $ ,$\alpha $ respectively. The filled circle represents the presence of the two photon input channels and the unfilled circle represents the block of the photon input channel$\beta $ ; (b) Entanglement and disentanglement of NOON state path using metasurface. (c) Signal photon imaging using entangled photon pairs in the above two figures, and different polarization measurements of idle photon can clearly distinguish the triangular and star patterns; the two images below are imaged using mixed photon pairs and cannot clearly distinguish between the two patterns; (d) Experimental setup for quantum weak measurement using a metasurface超构表面同样可以应用于量子测量[134],如图9(c)所示,具有正交线偏振态的纠缠光子对的量子态表示为
$\left| {{1_{\rm{H}}}} \right\rangle \left| {{1_{\rm{V}}}} \right\rangle$ ,当其以圆偏振态基矢表示时,其量子态表示为${{\left( {\left| {{2_{\rm{L}}}} \right\rangle \left| 0 \right\rangle - \left| 0 \right\rangle \left| {{2_{\rm{R}}}} \right\rangle } \right)} / {2{{i}}}}$ ,这是一种NOON态。当其经过超构表面时,利用超构表面的几何相位,左、右旋偏振态的光子分别出射到不同的方向。基于此原理,Georgi等人将超构表面与其他光学器件相结合构建了一个干涉仪,在其中一个路径放置一个相位片,可以发现,通过调控相位片引入的相位,符合计数呈现周期性振荡,这充分证明了纠缠光子对经过超构表面后依然保持很好的相干性,即该NOON态不同组成成分之间的相位差固定。此外,超构表面除了能够对量子态进行投影测量,还能够对量子态进行弱测量。量子弱测量作为量子测量一个重要的分支,首先提出于电子系统,通过给电子施加微弱的磁场,可以对电子的自旋量子态进行弱测量。它的主要思想是能够在不破坏量子态的前提下,获取量子态的信息。量子弱测量已经被用于波函数测量以及微弱信号放大等方面。类比于电子系统,在光学系统里,超构表面的相位梯度相当于电子系统的磁场,通过在超构表面上设计小的相位梯度,可以实现对光子量子态的弱测量[135]。如图9(d)所示为利用超构表面进行弱测量的实验装置图,GLP1(格兰激光偏振棱镜)用于制备光子的初态,超构表面提供微小的相位梯度,四分之一玻片和GLP2用于光子末态的后选择。通过选择不同的末态,即改变四分之一玻片与y轴的角度$\beta $ 和GLP2与x轴的角度$\alpha + \beta $ ,如图所示,当$\alpha = 0$ 时,弱值为纯虚数,随着$\beta $ 角度增大,光斑中心位置的位移增大,但是随着CCD位置的变远,光斑中心位置没有变化,这证明了纯虚数弱值对应着光子波函数的位置变化。当$\beta = 0$ 时,弱值为纯实数,随着$\alpha $ 角度增大,光斑中心位置的位移增大,并且随着CCD位置的变远,光斑中心位置逐渐增大,这证明了纯实数弱值对应着光子波函数的动量变化。 -
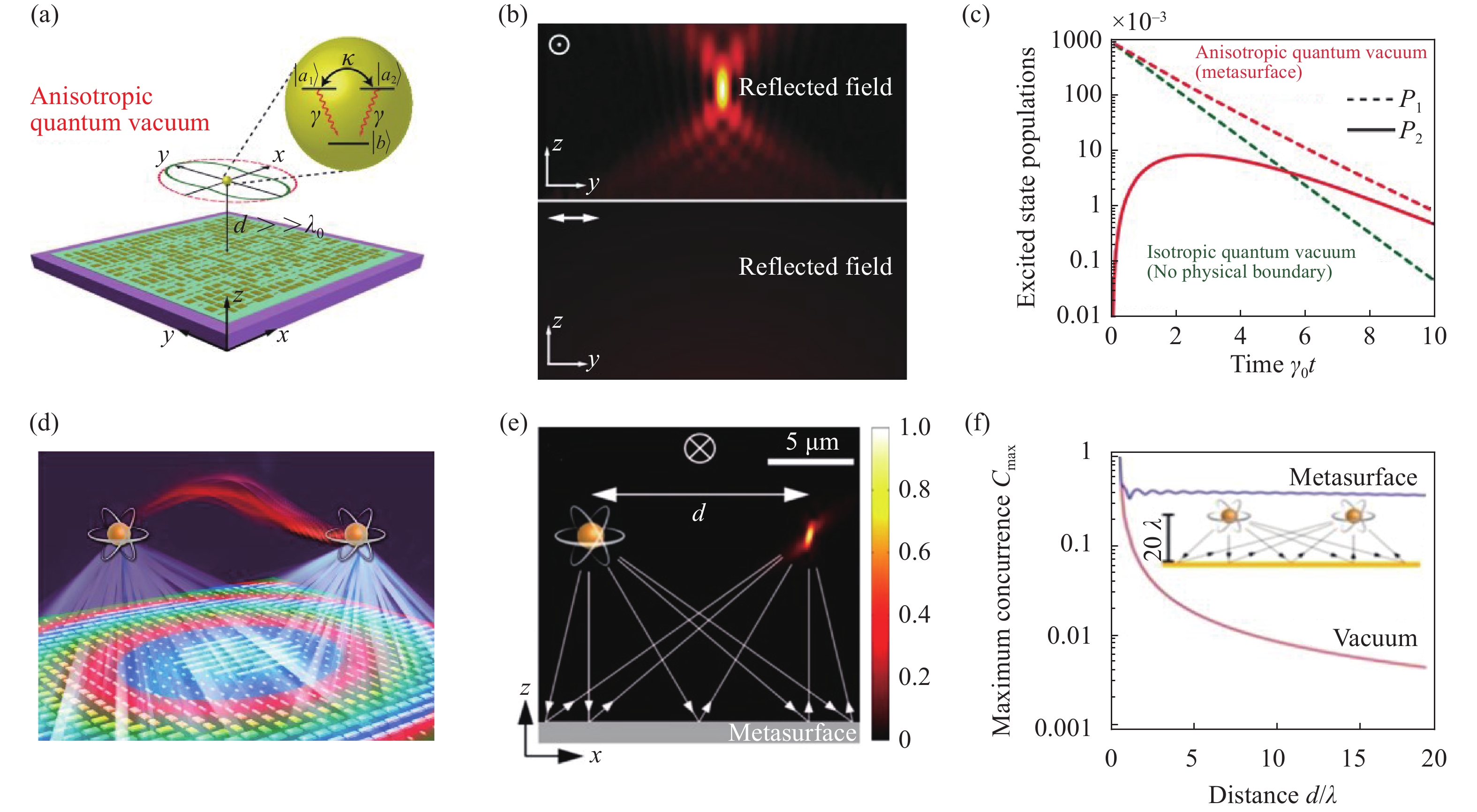
最初,人们认为量子发光体的寿命是其内禀性质,不受外界环境影响。直到Purcell提出量子发光体的寿命是可以被调控的,通过调控量子发光体周围的电磁场环境,量子发光体的辐射速率以及非辐射速率可以受到抑制或增强。基于此原理,研究者们设计了模式体积小、Q值高的结构,分别在空间和时间上增强量子发光体与光学结构的相互作用。在弱耦合领域,量子发光体的荧光寿命受到调制。在强耦合领域,量子发光体的能级与光学结构的能级发生杂化,在吸收谱、散射谱或者荧光谱上可以观察到拉比劈裂[136]。这些工作都是在光学结构的近场范围里对量子发光体的辐射行为进行调控,量子发光体位置的精确控制要求以及量子发光体靠近金属微纳结构的高损耗等因素限制了其发展。为了解决这些问题,在光学结构的远场范围里实现对量子发光体辐射行为的调制显得尤为重要。超构表面由于其出色的相位调控能力可以实现这一目的。Jha等人系统地研究了基于超构表面对于量子发光体的量子真空调控[137-139]。如图10(a)所示,超构表面打破了量子发光体的量子真空对称性,使得量子发光体的多个能级发生量子干涉,这在自由空间中是被禁止的[137]。通过合理地设计超构表面,使其对源点处电偶极子辐射的响应具有偏振依赖性,如图10(b)所示,即沿着x方向的电偶极子辐射的电磁场能够沿原路返回聚焦到源点处,最大效率为81%,沿着y方向的电偶极子辐射的电磁场没有这种效应。从量子力学角度来说,具有该种功能的超构表面打破了量子发光体的量子真空对称性,使得多能级量子发光体的不同能级能够发生量子干涉。如图10(c)所示,当没有超构表面时,
$\left| {{a_1}} \right\rangle $ 能级单指数衰减,$\left| {{a_2}} \right\rangle $ 能级占据数为0;当超构表面存在时,$\left| {{a_1}} \right\rangle $ 能级衰减速率变慢,$\left| {{a_2}} \right\rangle $ 能级占据数先增大后减小。这表明,超构表面引入的各向异性量子真空导致了$\left| {{a_1}} \right\rangle $ 能级占居数向$\left| {{a_2}} \right\rangle $ 能级的转移。进一步地,当考虑两个量子发光体时[138],如图10(d)~(e)所示,通过超构表面使得源点电偶极子辐射的电磁场能够定向地聚焦于目标电偶极子的位置,效率最高为82%,使得两个量子发光体产生纠缠。如图10(f)所示,当超构表面存在时,两个量子发光体的并发性比没有超构表面时增强两个数量级,其中并发性衡量两个量子发光体的纠缠度,随着两个量子发光体之间距离增大,当没有超构表面时,并发性快速下降,当存在超构表面时,在很长的距离依然保持很大的并发性。此外,还可以利用超构表面的几何相位调控单层过渡金属二卤化物的量子真空[139],实现激子自发的能谷间相干性。自由空间中,单层过渡金属二卤化物在布里渊区$K$ 、$K'$ 处简并的激子只能分别通过左旋、右旋偏振光激发,并且辐射的荧光分别对应为左旋、右旋偏振光。利用超构表面可以把源点处K谷激子辐射的左旋偏振光沿原路返回聚焦到源点处时,效率最高为47%,由于反射后左旋偏振光变成右旋偏振光,因此$K$ 谷激子可以间接激发$K'$ 谷激子,反之亦然。在超构表面调控量子发光体的量子真空的基础上,通过调节量子发光体的量子化轴线还可以实现光学活性[140]。此外,量子发光体的能级结构对于实验上实现超构表面的量子真空调制具有重要的意义。因为$V$ 型量子发光体具有两个激发态,一个基态,$\Lambda $ 型量子发光体具有一个激发态、两个基态,相对于$V$ 型量子发光体而言,$\Lambda $ 型量子发光体能级间具有更长的相干时间[141]。
Figure 10. Quantum vacuum engineering based on metasurface. (a) Based on the metasurface, the quantum vacuum symmetry of the quantum emitter is broken, so that quantum interference between different energy levels of the multi-level quantum emitter occurs. (b) Electromagnetic field radiated by an electric dipole in the x direction above the metasurface can return to focus to the source point along the original path, with the maximum efficiency being 81%, and the electromagnetic field radiated by an electric dipole in the y direction has no such effect; (c) When there is no metasurface, the energy level
$\left| {{a_1}} \right\rangle $ decayed exponentially and the energy level$\left| {{a_2}} \right\rangle $ occupied 0; when the metasurface exists, the decay rate of the energy level$\left| {{a_1}} \right\rangle $ decreases, and the energy level population$\left| {{a_2}} \right\rangle $ first increases and then decreases; (d) Schematic diagram of quantum entanglement of two quantum emitters based on metasurface; (e) Metasurface enables the electromagnetic field radiated by the source point electric dipole to be oriented to the position of the target electric dipole with the highest efficiency 82%; (f) Concurrence of two quantum emitters varies with the distance between them. The red solid line corresponds to the vacuum condition, and the blue solid line corresponds to the metasurface condition -
文中分别总结了超构表面在非线性光学以及量子光学方面的应用。从超构表面的非线性增强开始,分别介绍了金属等离激元微纳结构对于非线性频率转换过程的增强,金属超构表面的排布对于非线性频率转换效率的影响,基于高介电材料米共振的非线性增强,以及介质超构表面对于非线性频率转换过程的增强。值得一提的是,近年来,基于超构表面的准BIC模式和拓扑光学结构吸引了广大研究者越来越多的注意力,准BIC模式提供了一种在介质体系实现高Q值模式的新思路。拓扑超构表面将非线性过程与拓扑光学结合起来,为实现非线性频率转换过程的稳定增强、调控以及高效的收集提供了新的平台。超构表面因其出色的相位调控能力,在经典光学领域展示了非常广泛的应用前景。最近发现,超构表面也可以被应用于量子光学领域,文中依次介绍了基于超构表面的量子光源,基于超构表面的量子态调控,超构表面在量子光学相关方面的应用以及超构表面对量子发光体的量子真空调控。相对于传统光学器件而言,超构表面提供了更多的自由度用于制备和调节量子态,笔者等相信超构表面在量子光学领域的潜力还没有得到充分挖掘,例如利用超构表面收集高维度的纠缠光子并实现稳定、快速的高维度量子态测量,以及利用超构表面产生和调控轨道角动量纠缠的光子对。这对于量子通信、量子计算、量子测量等领域具有重要的意义,可能的应用场景包括高维量子信息编码以及模式复用光通信。
Nonlinear optics and quantum optics based on metasurface
doi: 10.3788/IRLA20201028
- Received Date: 2020-06-08
- Rev Recd Date: 2020-08-13
- Available Online: 2020-09-22
- Publish Date: 2020-09-22
-
Key words:
- metasurface /
- nonlinear optics /
- quantum optics
Abstract: In recent years, metasurface has received extensive attention in the field of classical light control, and excellent results have been obtained. At the same time, the application of metasurface in nonlinear optics and quantum optics has also attracted more and more interest. The basic principles and applications of nonlinear metasurface and quantum metasurface were introduced, and the related reports in recent years were summarized, including the harmonic generation and enhancement, the relationship between harmonic generation and symmetry, the nonlinear phase control and holography, and the generation, measurement and manipulation of entangled photon based on metasurface. Finally, the potential application of metasurface in these two fields were prospected.





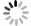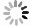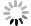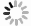






















































































 DownLoad:
DownLoad: