HTML
-
飞秒激光器诞生以来,因具有超短脉冲宽度、超宽带相干光谱、超高峰值功率等特性,被广泛地应用在超快成像[1-3]、极端物理环境产生[4-5]、光与物质相互作用[6]等前沿领域。除了窄脉冲、宽光谱特点外,飞秒激光器的低定时抖动特性在其应用中也起到了十分重要的作用,尤其是为低噪声光学频率梳的构建奠定了基础,极大地推动了高精度绝对距离测量、精密光谱测量、精密光学频率测量等领域的发展。
如今,飞秒激光器产生的超低定时抖动脉冲序列在高速数模转换[7]、大科学装置(如X射线自由电子激光器[8]、超快电子源[9])的定时同步、低噪声微波源[10]、相干脉冲合成[11]、激光雷达[12]等领域的应用也逐渐被开发出来,使之成为前沿科学研究中一种重要的新兴光源。文中将简要介绍飞秒激光器的定时抖动相关概念、测量方法以及各类飞秒激光器的定时抖动性能,并对其未来应用领域进行展望。
-
定时是指某件事情发生的特定时间点。通常电磁波脉冲的定时信息可以通过脉冲的时间重心(center of gravity, COG)来定义,其表达式为[13]:
式中:
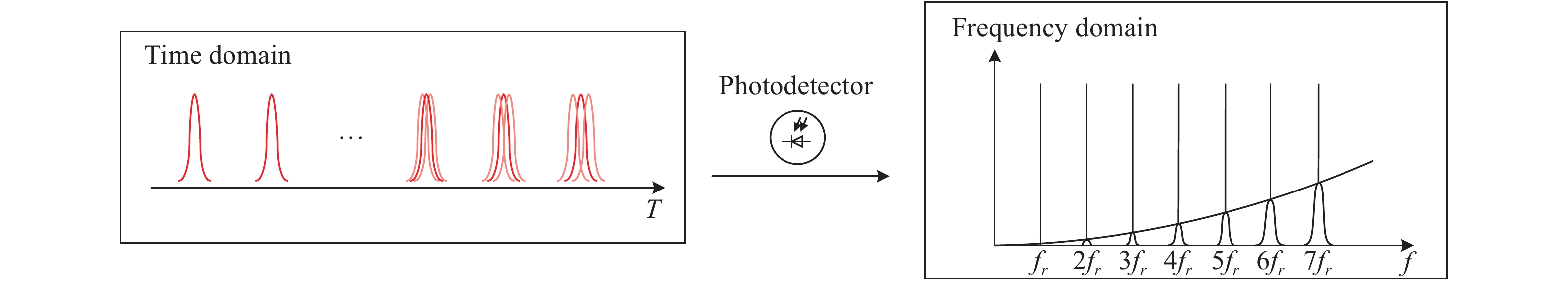
${{E}}\left({{t}}\right)$ 为脉冲的电场;${{t}}$ 为脉冲变化的时间。对于周期为${{T}}$ 的电磁波脉冲序列,均方根误差$\left\{{{{T}}}_{{n}}-{{n}}{{T}}|{{n}}= {1,2},3\cdots \right\}$ 称为定时抖动(Timing Jitter),用来描述脉冲的各个有效瞬时位置对其当时的理想位置的短期性随机偏离。在锁模激光器中,定时抖动定义为输出光脉冲包络与理想位置的脉冲包络线之间的时间偏差。这种时域上的抖动,在频域对应着脉冲重复频率的噪声。对于自由运转的锁模激光器来说,脉冲时序会无限制地发散并经历随机游走,如图1(a)所示。
在飞秒激光器的低抖动特性被广泛认同前,时钟信号源大都基于微波器件,通过射频波段的高频率载波实现授时。在微波信号源中,由于热噪声及谐振腔损耗的存在,其输出的载波信号的零点位置相对于理想周期性位置会有一定程度偏差,此偏差即为微波信号源的定时抖动。微波信号源的定时抖动,通常用衡量时间偏移量变化速率的扩散系数来表征:扩散系数越大,说明在一定的积分时间内,时间偏移量越大,定时抖动的水平也就越高。一般地,微波信号源定时抖动的扩散系数为[14]:
式中:
$ {T}_{0} $ 为载波信号的周期;$ k $ 为玻耳兹曼常量;$ T $ 为绝对温度,$ {E}_{mode} $ 为腔内振荡模式的能量;$ {\tau }_{cav,RF} $ 为腔内能量的衰减时间常数。与微波信号源相对应的,飞秒激光器中以放大自发辐射噪声(ASE)直接耦合至双曲正割形脉冲的定时抖动的扩散系数可以写成如下形式[14]:式中:
$ {\tau }_{p} $ 为脉冲的半极大全宽;$ {E}_{p} $ 为单脉冲能量;$ hv $ 为单光子能量;${\tau }_{cav, \; ML}=\dfrac{{T}_{rt}}{2{g}_{s}}$ 为腔内损耗时间常数;$ {g}_{s} $ 为饱和增益。对于工作在室温下、载波频率为10 GHz的微波信号源来说,$ {T}_{0} $ 为100$\rm{ps}$ ,$kT\approx 0.025\;\rm{eV}$ ;对于工作在室温下、中心波长为1040 nm的飞秒激光器,$hv\approx 1.2\;\rm{eV}$ ,$ {\tau }_{p} $ 约为100$\rm{fs}$ 。若假设二者的腔内模式能量与脉冲能量和衰减时间常数乘积位于同一数量级,则微波信号源的扩散系数约为飞秒激光器的$ {10}^{5} $ 倍。不难看出,飞秒激光器的低定时抖动的优势主要来源于其极窄的脉冲宽度。这种现象可以解释为:在飞秒激光器腔内,脉冲能量集中在飞秒量级的超短脉冲内,单位时间内的光子密度得到了极大的提升;对于一定的自发辐射(ASE)噪声水平,脉冲光子密度的提高可以降低ASE噪声引起的脉冲时域分布的相对变化量,进而降低其对于脉冲时域位置的短期稳定性的干扰,使得脉冲的定时抖动保持在极低水平。 -
锁模激光器的定时抖动在时域表现为脉冲实际位置对理想位置的偏移,在频域上则与重复频率的相位噪声相对应,如图1(b)所示。
得益于微波器件相位测量技术的快速发展[15-16],飞秒激光器的定时抖动可以通过使用低噪声光电二极管来进行直接测量。光电探测器可以将飞秒激光器输出的光脉冲转化为射频波段的电压信号,然后使用信号分析仪来检测激光器重复频率高次谐波的相位噪声[17]。
这种方法结构简单、技术成熟,在飞秒激光器定时抖动研究初期起到了至关重要的作用。但是随着研究的深入,这种方法的局限性逐渐显露:首先,飞秒激光器输出脉冲的脉冲宽度一般在百飞秒量级甚至更窄,对应着10太赫兹(
$ {\rm{T}}{\rm{H}}{\rm{z}},{10}^{12}\;{\rm{H}}{\rm{z}} $ )以上的带宽,而目前商用的光电探测器的带宽往往在百吉赫兹($ {\rm{G}}{\rm{H}}{\rm{z}}, {10}^{9}\;{\rm{H}}{\rm{z}} $ )以内,因此光电转换的过程会不可避免地引入低通滤波效应,损失掉飞秒激光器的高时间分辨率的优势。其次,飞秒激光器输出脉冲的定时抖动一般在飞秒或者亚飞秒量级,远比一般的微波信号源噪声水平低,因此测量过程对系统中的光电探测器、信号分析仪以及频率基准的自身噪声水平提出了更高的要求,精确测量的复杂程度和成本也大幅度增加。尤其是光电二极管的强度噪声向相位噪声的耦合系数较高,因此测量时的光电转换过程将会引入过高的相位噪声。从以上分析不难看出,直接使用光电探测器来测量飞秒激光器的定时抖动,测量的分辨率受到了微波器件的限制,测量的动态范围也相应降低。因此,早期的飞秒激光器定时抖动的测量结果一般在10 fs量级[16-19]。
-
为了进一步提高飞秒激光器定时抖动的测量分辨率,利用平衡光学互相关来测量定时抖动的方法被提出并逐渐普及。这种方法使用平衡光学互相关器来代替了光检测和混频器件。由于飞秒激光脉冲本身具有很高的定时鉴别灵敏度,可以确保BOC方法具有亚飞秒甚至阿秒级的时间分辨率。因此,可以实现超高的定时抖动测量灵敏度和宽动态范围[20-24]。
基于BOC的定时抖动测量系统如图2所示。为了解决飞秒激光器定时抖动低于微波器件本身底噪的问题,该方法采用了另一台与被测激光器具有相同或者更低定时抖动水平的飞秒激光器作为本振激光器。平衡互相关信号的产生基于两个正交偏振光在晶体中传输的群延迟差以及二类相位匹配的非线性和频效应。如图2所示,具有
$ {\Delta t} $ 相对时间延迟的正交偏振的飞秒激光脉冲合束后入射至互相关器中。脉冲首先透过一面双色镜并聚焦至非线性晶体。两个正交偏振态的脉冲经过晶体时由于群速度失配会发生一个脉冲对另一个脉冲的赶超,在此过程中伴随和频信号的产生。非线性晶体之后放置另一面双色镜,使得和频信号透过双色镜,并由平衡探测二极管的一个端口探测。基频信号经双色镜反射再次聚焦至晶体,产生的和频信号由平衡探测二极管的另一个探测头探测。平衡探测器的输出电压值只与入射至互相关器的两个脉冲的时间间隔有关,而激光脉冲的强度波动引起的相关信号由平衡探测过程完全抵消。在不同的初始时间延迟$ {\Delta t} $ 下,两个基频光在非线性晶体中的重叠程度不同,将产生不同功率的和频信号,并由两个光电二极管转化为不同的电压值。典型的平衡探测器的输出的电压值随入射脉冲时间间隔$ {\Delta t} $ 的变化如图2的插图所示。常用光学互相关信号的功率谱密度(PSD)来表征飞秒激光器的时域稳定性。BOC方法中测得的定时抖动功率谱是两台激光器的总和。由于两台激光器的定时抖动水平相似且在锁定带宽之外各自独立运行,因此将测量结果除2就可以得到待测激光器对应的平均定时抖动。
利用平衡光学互相关技术测量定时抖动的优势在于:它提供了比传统的微波混频手段更高的时间探测精度且不受限于额外的光电探测引入的相位噪声。另外,平衡探测器可以消除激光器强度噪声对测得的定时抖动结果的影响,使测量信号仅与时间信息有关。当两个激光器的重复频率用低带宽的伺服环路锁定时,残留的定时抖动就可以通过光学互相关信号进行探测。
尽管BOC方法已经成功地将飞秒激光器的定时抖动测量结果降低至了阿秒量级,但它在广泛普及上仍有许多困难。首先,对两台飞秒激光器的重频进行锁定会使得实验装置十分复杂,测量成本增加。其次,由于该方法的时间分辨率是由超短脉冲的持续时间决定的,而当被测激光器的脉冲宽度较长(数百飞秒至皮秒)时,测量的动态范围也会相应地受到限制。
-
另一种高分辨率的定时抖动测量方法是科罗拉多大学的侯东等人提出的基于光学外差探测的定时抖动测量技术[25]。该方法是通过将两台激光器输出的不同频率,如
$\left(m{f}_{rep}+{f}_{ceo}\right)$ 和$ \left(n{f}_{rep}+{f}_{ceo}\right) $ 处,分别做拍频,并将两个信号混频,这样可以消除两台激光器的相对载波-包络偏移频率的影响,进而得到只与两台激光器重复频率差有关的信号。实验证实,这种测量方法的本底噪声约为$2.8\times {10}^{-13}\;\rm{fs}^{2}/\rm{H}{z}$ [25],比BOC方法获得的结果[26]低约10 dB,可作为BOC方法的替代方法之一。 -
不论是平衡光学互相关法还是光学外差法,在测量激光器的定时抖动时都需要引入参考激光器。为了解决这一问题,韩国KAIST的J. Kim等人提出了一种基于非对称放置的光纤延迟线的干涉仪来测量激光器定时抖动的方法[27]。该方法使用公里级长的光纤延迟线对激光器输出的脉冲进行延迟,将延迟后的脉冲与激光器直接输出的脉冲进行比较,即可测得激光器的定时抖动。天津大学田昊辰等人在此基础上,搭建了一种基于非对称光纤延迟线干涉仪的复合测量平台[28]。该装置可以对飞秒激光频率梳的定时抖动、载波包络偏移频率噪声和梳齿线宽进行测量,降低了测量成本,更加实用。
-
前述方法对定时抖动的表征主要通过测量激光器的定时抖动噪声功率谱密度来进行,它们能够精确地给出定时抖动的在频域的频率分布情况及噪声特性,方便与理论分析进行对照。但在光通信等实际使用领域中,常常使用“眼图”来表示信号在时域中的定时抖动的水平和分布,具有简单直观的优势。然而,由于飞秒脉冲宽度极窄,电子学的“眼图”分析方法不再适用。为了解决这一问题,天津大学的师浩森等人提出了一种基于异步光学采样[29-30]方法的定时抖动分析法,可以利用示波器等电子学设备对飞秒激光脉冲序列的亚飞秒的定时抖动进行“可视化”的实时分析。
基于异步光学采样的定时抖动时域分析原理图如图3所示。系统包含一台待测激光器和一台本振激光器,重复频率分别为
$ {f}_{r} $ 和$ {f}_{r}-\Delta {f}_{r} $ 。对于无定时抖动的理想情况,如图3(a)中所示,由于两台激光器具有微小的重复频率差$ \Delta {f}_{r} $ ,因此在每个重复频率周期内,本振光脉冲会对待测光脉冲的不同位置进行采样并转化为电信号。在经历$N=\dfrac{{f}_{r}}{\Delta {f}_{r}}$ 个本振光脉冲后,可以完成对待测激光脉冲的一次采样,所用时间为${T}_{r}=\dfrac{1}{\Delta {f}_{r}}$ 。因此,异步光学采样法可以将飞秒量级的光脉冲在时域拉伸$ N $ 倍,转化为较慢变地、易测量的电脉冲,只需要确定放大倍数$ N $ 就可以实现亚飞秒甚至阿秒级的定时分辨率。
Figure 3. Principle of time-domain timing jitter characterization method based on ASPOS, the ASOPS process (a) without LUT timing jitter (b) with LUT timing jitter
在使用该方法进行定时抖动表征时,如图3(b)所示,异步光学采样过程不仅在时域上拉伸了飞秒激光脉冲,而且还同时放大了定时抖动,即采样得到的电脉冲位置将包含定时抖动的信息。使用正确的触发将所用的脉冲与前脉冲对齐,则后一周期的电脉冲位置将产生抖动,称为表观周期抖动。因此,对电脉冲信号的表观周期抖动进行眼图分析,即可将其映射至光脉冲的实际的周期抖动[30],从而实现对激光器定时抖动的高时间分辨率测量。
-
随着飞秒激光器定时抖动理论的不断完善和测量精度的逐渐提升,关于低抖动的飞秒激光源的实验研究也逐渐展开。
固体飞秒激光器由于输出脉冲具有极窄的脉冲宽度和极高的峰值功率,ASE直接耦合的定时抖动水平较低。目前为止,飞秒激光器低定时抖动的纪录是麻省理工大学的A. J. Benedick等人通过BOC方法在脉冲宽度为10 fs的钛宝石飞秒激光器内获得的13 as的残余定时抖动[22]。另外,在基于SESAM锁模的Cr:LiSAF激光器和Er:Yb-glass激光器中,也分别获得了30 as[31]和83 as[32]的定时抖动(积分带宽为[10 kHz-50 MHz])。然而,由于固体激光器往往对腔镜的空间耦合精度要求极高,且使用和维护成本较高,因此很难在实验室以外的环境大范围地推广应用。
与固体激光器相比,光纤激光器的光-光转化效率更高,热效应不明显,结构紧凑,价格低廉,更具有实用化优势。近年来,光纤飞秒激光器的定时抖动性能被不断优化,已经可以接近固体飞秒激光器的参数,进一步提高了其实用价值。2007年,韩国KAIST的J. Kim等人首次利用BOC技术实现了对掺铒光纤激光器的高频抖动的测量,在[10 kHz,10 MHz]积分带宽内获得了5 fs的定时抖动[33]。之后,宋有建等人在实验中探索了腔内净色散与脉冲序列定时抖动的耦合机制,通过优化激光器的色散参数将掺镱和掺铒光纤激光的高频定时抖动分别降低至175 as[20]和76 as[26]。2014年,天津大学的秦鹏等人通过在腔内加入窄带滤波器,使得掺镱激光器在很宽的腔色散范围内均可获得较低的的定时抖动[23],降低了低抖动飞秒激光源设计的难度。由于上述激光器均为基于非线性偏振旋转(Nonlinear Polarization Evolution, NPE)锁模的飞秒激光器,因此对于环境扰动和光纤应力较为敏感。2016年,美国IMRA公司N. Kuse等人设计了的一种基于非线性放大环形镜(Nonlinear Amplifying Loop Mirror, NALM)锁模的全保偏9字腔激光器,可获得40 as的定时抖动[34]。其研究提高了激光器长期稳定性和可重复性,为低定时抖动光纤飞秒激光器的实用化奠定了基础。
尽管上述工作在降低锁模固体飞秒激光器和光纤飞秒激光器的定时抖动方面取得了巨大进步,但是这些阿秒级定时抖动性能的激光器重复频率大多被限制在100 MHz。为了探究高重频飞秒激光器的定时抖动特性,麻省理工大学的J. Chen等人通过在200 MHz激光振荡器外加入自由空间Fabry-Perot腔作为重频倍增器,获得了重复频率为2 GHz,定时抖动为27 fs的脉冲[35]。韩国KAIST的H. Yang等人搭建了重复频率为1.13 GHz的Yb:KYW固体激光器,并测得其在[17.5 kHz,10 MHz]带宽内的定时抖动为0.7 fs[36]。北京大学的王燕等人测量了基于NPE锁模的掺Yb光纤激光器在重复频率为882 MHz时的定时抖动,在[30 kHz,5 MHz]的积分带宽内最低为10 fs[37], 并提出高重频的光纤飞秒激光器的定时抖动可以通过减小相对强度噪声与定时抖动的耦合来抑制[38]。西安光机所的张建国等人报道了一种2.68 GHz全光纤SESAM锁模光纤激光器,在[300 Hz,30 MHz]的积分带宽内测得的定时抖动为82.5 fs[39]。此外,半导体锁模激光器和微环谐振腔也是可以产生数十GHz高重频超短脉冲的可靠光源。但是,由于半导体锁模激光器的脉冲宽度通常在皮秒量级,很难实现飞秒量级以下的极低抖动[40-42]。2020年,韩国KAIST课题组的D. Jeong等人测量了一台22 GHz的硅基微环光疏的定时抖动,在[10 kHz, 3 MHz]积分带宽内获得了2.6 fs的定时抖动积分值[43],进一步证明了微环谐振腔作为高重频低抖动飞秒激光光源的应用潜力。
除单脉冲运转状态之外,飞秒激光器还能工作在多脉冲的束缚态。德国马普所的庞盟等发现,利用光机械力可以束缚住激光器内多个孤子,并编程调控其间隔,孤子之间的抖动小于100 fs[44],这种稳定的多孤子状态能够在激光器内一直稳定运转,为全光存储提供了一种新的思路。天津大学的师浩森等人发现,激光器内,光孤子尾部相互作用产生的紧束缚态孤子对的定时抖动<1 fs[45],这种稳定的双孤子结构有可能为全光信息处理提供一种新型多级字母表编码单元(multi-alphabet coding unit)。
此后,基于具有极限分辨能力的测量手段与激光腔参数的优化,不同结构的低定时抖动的飞秒激光器层出不穷,为其在前沿领域的广泛应用奠定了坚实的基础[46]。表1列举了近年来低抖动飞秒激光器的相关研究进展。表2展示了目前商用飞秒激光器的定时抖动水平。
Laser source Integrated
timing jitterIntegrated Fourier
frequency rangeMeasurement
methodResearch
instituteClassification Laser cavity parameter Er-doped
fiber laser194-MHz NPR,soliton mode-
locking regime (2007)[47]18 fs [1 kHz–10 MHz] PD MIT 3-GHz SESAM,soliton mode-
locking regime (2009)[48]19 fs [10 kHz–40 MHz] PD MIT 200-MHz NPR with spectral filter,stretched-pulse mode-locking regime (2010)[49] 17.4 fs [1 kHz–10 MHz] PD Shanghai Jiao Tong University 1-GHz SESAM,soliton mode-
locking regime (2010)[50]22 fs [1 kHz–10 MHz] PD MIT 80-MHz NPR,cavity dispersion
$-0.002\;\rm{ps}^{2}$ (2011)[26]0.07 fs* [10 kHz–40 MHz] BOC KAIST 80-MHz CNT-SA,cavity dispersion
$-0.02\;\rm{ps}^{2}$ (2013)[51]0.5 fs [10 kHz–40 MHz] BOC KAIST 463-MHz MoS2-SA,soliton mode-
locking regime (2015)[52]33 fs [1 kHz–1 MHz] PD Shanghai Jiao Tong University 80-MHz NPR,cavity dispersion
$+0.002\;\rm{ps}^{2}$ (2015)[53]0.7 fs [10 kHz–10 MHz] BOC KAIST 129-MHz NPR with spectral filter,cavity dispersion$+0.008\;\rm{ps}^{2}$ (2015)[54] 3.46 fs [10 kHz–10 MHz] BOC KAIST 75-MHz NALM,cavity dispersion
$-0.003\;\rm{ps}^{2}$,soliton molecule (2018)[45]0.83 fs [10 Hz–2 MHz] BOC Tianjin University 36.56 MHz NALM,soliton mode-
locking regime (2019)[55]7.3 fs [10 kHz–1 MHz] FDL KAIST 2.68 GHz SESAM,cavity dispersion
$\sim 50\;\rm{fs}^{2}$ (2019)[39]82.5 fs [300 Hz-30 MHz] PD Xi'an Institute of Optics and Precision Mechanics of CAS Yb-doped
fiber Laser80-MHz NPR,stretched-pulse mode-
locking regime (2011)[20]0.18 fs* [10 kHz–40 MHz] BOC KAIST 80-MHz NPR,soliton laser (2011)[20] 1.8 fs [10 kHz–40 MHz] BOC KAIST 80-MHz NPR,amplifier-similariton (2011)[20] 2.9 fs [10 kHz–40 MHz] BOC KAIST 80-MHz, NPR with spectral filter,cavity dispersion $+0.008\;\rm{ps}^{2}$ (2014)[23] 0.57 fs [10 kHz–10 MHz] BOC Tianjin University 10-MHz SESAM,all-PM,cavity dispersion $+0.46\;\rm{ps}^{2}$ (2016)[24] 5.9 fs [10 kHz–5 MHz] BOC Tianjin University 880 MHz NPR,stretched-pulse mode-
locking regime (2018)[37]10 fs [30 kHz–5 MHz] BOC Peking University Tm-doped
fiber laser690-MHz harmonic mode-locking NPR,soliton mode-locking regime (2015)[56] 6 ps [100 Hz–1 MHz] PD Tsinghua University 253 MHz SBR Tm/Ho fiber laser (2016)[57] 20 fs [100 Hz–2 MHz] PD Boston University 1.5 GHz SESAM (2018)[58] 940 fs [10 Hz–1 MHz] PD South China University of Technology Solid
laser80-MHz KLM Ti:sapphire laser (2012)[22] 0.013 fs* [100 Hz–40 MHz] BOC MIT 100-MHz SESAM Cr:LiSAF laser (2012)[31] 0.03 fs [10 kHz–50 MHz] BOC MIT 100-MHz, SESAM, Er:Yb-glass laser (2013)[32] 0.083 fs [10 kHz–50 MHz] BOC CSEM 500-MHz SESAM Er:Yb-glass laser (2015)[25] 0.016 fs [10 kHz–250 MHz] OH University of Colorado Semiconductor
laser10 GHz SCOWA-based harmonically mode-locked nested cavity laser (2018)[59] 3.4 fs [10 Hz,100 MHz] PD University of Central Florida 21 GHz InAs/InP quantum dash laser (2018)[41] 400 fs [10 kHz,100 MHz] PD University College Cork 19 GHz integrated heterogeneous silicon/III-V colliding pulse mode-locked laser (2018)[42] 1.2 ps [100 kHz,100 MHz] PD University of California Optical
Microresonator22 GHz silica microcomb (2020)[43] 2.6 fs* [10 kHz–3 MHz] FDL KAIST 注:*表示该类激光器测得的最低定时抖动值
* indicates the measured lowest timing jitter of lasers in this categoryTable 1. Representative progress of low timing jitter femtosecond laser
Laser source Integrated timing jitter Integrated Fourier frequency range Company Figure 9 fiber laser <2 fs [10 kHz–10 MHz] Menlo System Figure 9 Er- fiber laser[34] 0.04 fs [10 kHz–10 MHz] IMRA America MENHIR-1550 ≤30 fs [1 kHz–10 MHz] MenHir Photonics ORIGAMI 5-40 <30 fs [1 kHz–10 MHz] NKT Photonics Table 2. Timing jitter of commercial femtosecond laser
-
精密定时是许多分布式大科学装置的必备技术。极具代表性的一个应用是目前世界各地在建的下一代X射线自由电子激光器(XFEL),包括美国斯坦福的硬X射线自由电子激光器(LCLS)[60]、德国的European XFEL[61]、瑞士的SwissFEL[62]、日本的SACLA[63]以及我国上海软X射线自由电子激光装置(SXFEL)[64]和上海硬X射线自由电子激光装置(SHINE)[65]等等。这些科学装置的设计目标是产生高能阿秒X射线脉冲[66],进而可以实现亚原子量级的空间分辨率,用来观测物理、化学反应的过程[67-68]。为了产生阿秒量级的X射线脉冲,需要对X射线自由电子激光器内部的所有光源和子微波源进行飞秒甚至阿秒精度的定时同步[13]。SwissFEL的定时同步系统中,德国Cycle GmbH, Notkestr等人使用了800 m长的单模光纤搭建了光纤链路来对激光器进行定时同步,该系统在[20 µHz,1 MHz]下获得了2.6 fs的抖动积分值[69]。在European XFEL的设计中,德国CFEL的辛明等人利用平衡光学互相关技术在3.5公里长的保偏光纤链路中对两台激光器进行了远程同步,40小时内的定时抖动仅为2.5 fs[70]。由于飞秒激光器输出的脉冲序列具有极低的高频定时抖动,因此可作为低抖动的时钟信号源和种子光振荡器,在这种现代大科学装置的建设中发挥至关重要作用。
-
低定时抖动的信号源在信息处理领域同样具有重要的作用。模拟信号到数字信号的高速、高精度转换,是现代信息处理最基础的环节。然而,现阶段常见的模数转换系统(Analog-Digital Converter, ADC)大多基于微波器件。尽管微波器件在理论和技术上都较为成熟,但是受到其高频定时抖动的限制,在较高的采样频率下,模数转换系统能够实现的有效采样位数十分有限[71]。由于现代芯片级时钟电路最低只可以达到100 fs的定时抖动,因此无法在10 GHz的采样频率下实现6位以上的有效采样位数。
为了兼顾高采样频率和高有效采样位数,必须降低采样信号源的定时抖动水平。飞秒激光器的高频定时抖动往往在飞秒甚至阿秒量级,将其作为采样信号进行模数转换,可以实现在高采样率下获得更高的有效采样位数。麻省理工大学的J. Kim等人通过将商用的掺镱飞秒激光器输出的超短脉冲序列用作采样信号,使用电光强度调制器对40 GHz的射频载波信号进行了采样实验。由于采样信号具有较低的定时抖动(在[10 kHz,2 MHz]的频率带宽内的定时抖动小于5 fs),该系统在2 MHz的探测带宽内的信噪比为44.3 dB,有效采样位数达到了7.06位[72]。上海交通大学的邹卫文等人构建的深度学习供电的光子模数转换(DL-PADC)体系结构中,使用了CALMAR公司的PSL-10-TT主动锁模激光器(实验测得定时抖动为26.5 fs)作为光子前端,来提供采样光脉冲序列和精确的量化时钟。在使用DL-PADC对23 GHz的载波信号进行采样时,有效采样位数可达9.24位。[21]
-
近年来,空间合成孔径、地形地貌测量以及大型工件制造等前沿应用的出现,对距离测量的精度、动态范围和更新速率等指标提出了更高的要求。而随着光纤飞秒激光器的发展,低成本、高集成化、低抖动的特性进一步推动了其在高精度绝对距离测量领域的应用,飞秒激光频率梳合成波长干涉测距、多波长干涉测距、飞行时间测距、光谱色散测距等测量方法被相继提出[73]。尤其是基于两台具有一定重复频率差的低噪声飞秒激光器实现的双光梳绝对距离测量技术[74-75]更是以其高更新速率、高精度和大量程的优势得到了广泛关注。
在双光梳绝对距离测量中,对脉冲飞行时间的测量精度是影响最终测距精度的一个主要因素。在理想情况下,飞秒激光输出的光脉冲序列为严格的周期信号,通过异步光学采样后可以得到稳定的采样电脉冲序列,然后通过电脉冲间的时间间隔解算出光脉冲的飞行时间,进而实现高精度的绝对距离测量。但在实际情况中,由于本振光脉冲与参考光脉冲序列之间存在着相对定时抖动,而异步采样过程会将两个光脉冲序列之间的定时抖动进行放大,并导致得到的采样电脉冲信号发生波动,最终造成距离测量上的误差。虽然通过多次平均可以降低测量结果的不确定度,但这会造成测量更新速度的下降,限制了双光梳测距的测量更新速率。因此,为了从根本上提高双光梳绝对距离测量的测距精度,需要极低抖动的飞秒激光器作为光源,同时进行合理设计,使激光器具有良好的环境稳定性,更适用于实际应用。天津大学的师浩森等人就定时抖动对双光梳绝对距离测量的影响进行了详细的理论分析,并通过实验证实,低抖动的飞秒激光源可以显著提升双光梳绝对测距的精度,并放宽双光梳稳频的要求[76]。
-
光束合成可以分为非相干合成和相干合成两大类。与非相干合成的强度叠加不同,相干合成中各路激光要实现稳定的干涉,进行振幅叠加。该技术能够克服单台光纤激光器功率限制以获得高输出功率,同时解决亮度、热管理等问题,保持良好的光束质量,是高功率激光技术的一个重要研究方向。早期的相干合成研究主要针对连续激光。国防科技大学马阎星等人通过主动相位控制对9路百瓦级光纤放大器进行相干合成,实现了1.08 kW的输出功率[77]。2017年,国防科技大学的刘泽金等人通过4路光纤激光的相干偏振合成,实现了5.02 kW的高功率输出[78]。
同样地,对于飞秒激光器来说,由于增益介质的增益带宽和掺杂浓度的限制,单台激光器很难直接获得超过一个倍频程的光谱和瓦量级的飞秒脉冲输出。而将两台飞秒激光器进行相干合成,可以扩展飞秒脉冲的光谱,提高飞秒激光的输出功率。
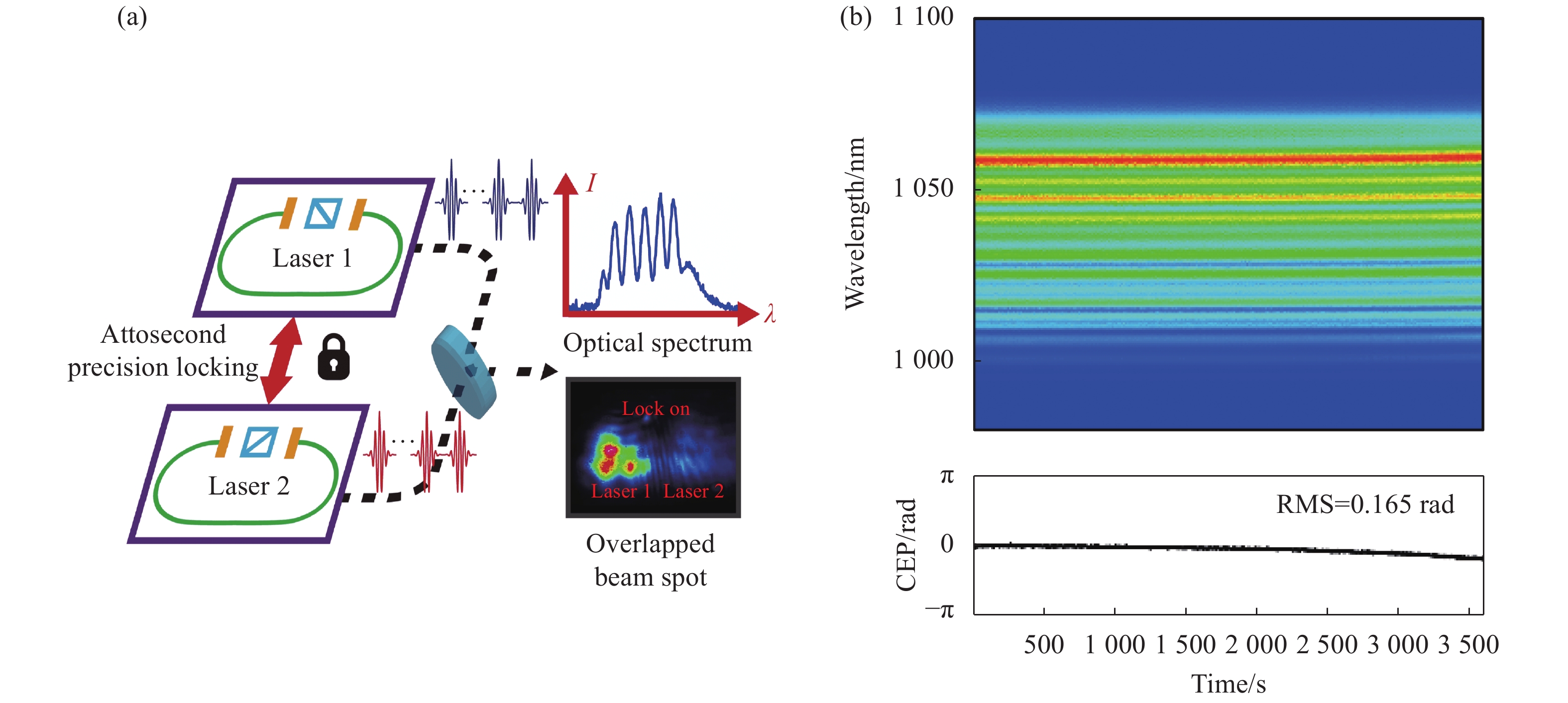
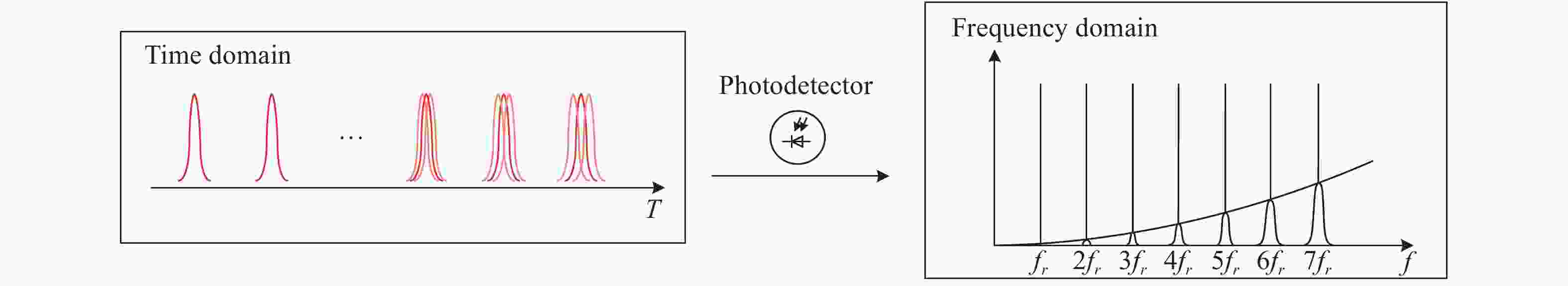
2001年,NIST的Sheldon等人首次证明了两台飞秒激光器相干脉冲合成的可能性[79]。然而,该技术的实现面临着巨大的挑战。一个必要的前提是两台飞秒激光器的相对定时抖动和载波-包络相位抖动必须稳定至数十阿秒,达到原子时间尺度[80]。因此,直到平衡互相关技术的产生和发展,才使得飞秒激光的相干合成成为可能。2012年,J. A Cox等人通过将钛宝石激光器和掺铒光纤激光器产生的超连续谱的相干合成,实现二者之间的剩余相对定时抖动为2.2 fs,得到了超过一个倍频程的光谱和脉冲宽度为3.7 fs的近单周期时域脉冲[81]。2016年,天津大学的田昊辰等人通过优化激光腔的量子噪声,对两台独立的掺镱光纤飞秒激光器进行相干合束,如图4所示,实现了长达1小时的稳定运转,克服了此前飞秒激光相干合成维持时间短、难以实用化的缺点,为单周期脉冲合成、光学任意波形产生及飞秒频率梳的远程分布等应用带来了突破[82]。2019年,天津大学的葛爱晨等人搭建的掺镱光纤飞秒激光器的相干合成系统,两个脉冲之间的相对定时抖动小于光周期的十分之一,在放大后利用光子晶体光纤进行非线性脉冲压缩,获得了8 fs的时域脉冲[83]。
-
与常用的微波信号源以及其他类型的脉冲激光器相比,飞秒激光器输出的超短脉冲序列在时域上具有极低的定时抖动;在频域上,则提供了一个处于射频频率且相位噪声极低的重复频率信号源。飞秒激光器的输出脉冲序列同时携带了低抖动的光频信号和射频信号,可以实现与现有的光学、射频系统的低成本对接。
随着飞秒激光器在低抖动方面的优势日益明显,关于其定时抖动的研究也逐渐开展:不仅定时抖动的理论体系逐渐完善,相关的测量手段也在不断进步,这些都为低抖动飞秒激光器的开发奠定了坚实的基础。与此同时,随着低抖动飞秒激光器的推广应用,一些全新的方法和技术也为其定时抖动水平的进一步优化提供了新的思路。比如,近期快速兴起的基于微腔孤子的光学频率梳[84-85],由于其具有极高的重复频率(通常在10 GHz~100 GHz以上)、较低的定时抖动水平[43]同时兼具易于微型光子集成、发展为芯片系统的潜力,无疑是未来激光雷达、精密测距、高速ADC领域的理想低抖动飞秒光源之一。
在各方面因素的共同促进下,低抖动飞秒激光器的研究已经进入了一个全新的时代,相关领域的飞速发展使其在未来应用中存在着无限的可能性。





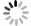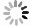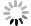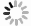








































 DownLoad:
DownLoad: